Advertisements
Advertisements
प्रश्न
Find the length of a chord which is at a distance of 4 cm from the centre of the circle of radius 6 cm.
उत्तर
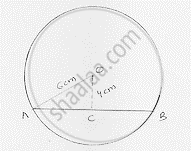
Radius of circle (OA)= 6cm
Distant (OC)=4cm
In Δ OCA by Pythagoras theorem
`AC^2+OC^2=OA^2`
⇒`AC^2+4^2=6^2`
⇒`AC^2=36-16`
⇒`AC=sqrt20 =4.47 cm`
WKT, the perpendicular distance from center to chord bisects the chord.
`AC=BC=4.47cm `
`Then , AB = 4.47+4.47`
`8.94 cm `
APPEARS IN
संबंधित प्रश्न
If a line intersects two concentric circles (circles with the same centre) with centre O at A, B, C, and D, prove that AB = CD (see given figure).

A circular park of radius 20m is situated in a colony. Three boys Ankur, Syed and David are sitting at equal distance on its boundary each having a toy telephone in his hands to talk each other. Find the length of the string of each phone.
true or false
A circle has only finite number of equal chords.
Find the length of a chord which is at a distance of 5 cm from the centre of a circle ofradius 10 cm.
In a circle with centre O, AB and CD are two diameters perpendicular to each other. The length of chord AC is
Two equal circles of radius r intersect such that each passes through the centre of the other. The length of the common chord of the circle is
If AB is a chord of a circle, P and Q are the two points on the circle different from A and B, then
In a circle of radius 17 cm, two parallel chords are drawn on opposite side of a diameter. The distance between the chords is 23 cm. If the length of one chord is 16 cm, then the length of the other is
Two chords AB and CD of a circle are each at distances 4 cm from the centre. Then AB = CD.
AB and AC are two chords of a circle of radius r such that AB = 2AC. If p and q are the distances of AB and AC from the centre, prove that 4q2 = p2 + 3r2.
