Advertisements
Advertisements
प्रश्न
If there were only one type of charge in the universe, then ______.
- `oint_s` E.dS ≠ 0 on any surface.
- `oint_s` E.dS = 0 if the charge is outside the surface.
- `oint_s` E.dS could not be defined.
- `oint_s` E.dS = `q/ε_0` if charges of magnitude q were inside the surface.
विकल्प
a and d
a and c
b and d
c and d
उत्तर
b and d
Explanation:
From Gauss’ law, we know `oint_s` E.dS = `q/ε_0`, here q is the charge enclosed by the closed surface. If `oint_s` E.dS = 0 then q = 0, i.e., net charge enclosed by the surface must be zero. If the charge is outside the surface, then charge enclosed by the surface is q = 0 and thus, `oint` E.dS = 0.
APPEARS IN
संबंधित प्रश्न
State and explain Gauss’s law.
A charge ‘q’ is placed at the centre of a cube of side l. What is the electric flux passing through each face of the cube?
Answer the following question.
State Gauss's law for magnetism. Explain its significance.
The surface considered for Gauss’s law is called ______.
Gauss' law helps in ______
The Electric flux through the surface
 (i) |
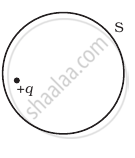 (ii) |
 (iii) |
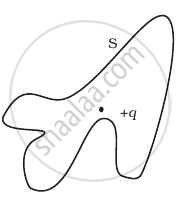 (iv) |
Consider a region inside which there are various types of charges but the total charge is zero. At points outside the region
- the electric field is necessarily zero.
- the electric field is due to the dipole moment of the charge distribution only.
- the dominant electric field is `∞ 1/r^3`, for large r, where r is the distance from a origin in this region.
- the work done to move a charged particle along a closed path, away from the region, will be zero.
If the total charge enclosed by a surface is zero, does it imply that the elecric field everywhere on the surface is zero? Conversely, if the electric field everywhere on a surface is zero, does it imply that net charge inside is zero.
In 1959 Lyttleton and Bondi suggested that the expansion of the Universe could be explained if matter carried a net charge. Suppose that the Universe is made up of hydrogen atoms with a number density N, which is maintained a constant. Let the charge on the proton be: ep = – (1 + y)e where e is the electronic charge.
- Find the critical value of y such that expansion may start.
- Show that the velocity of expansion is proportional to the distance from the centre.
The region between two concentric spheres of radii a < b contain volume charge density ρ(r) = `"c"/"r"`, where c is constant and r is radial- distanct from centre no figure needed. A point charge q is placed at the origin, r = 0. Value of c is in such a way for which the electric field in the region between the spheres is constant (i.e. independent of r). Find the value of c:
