Advertisements
Advertisements
प्रश्न
In a triangle, the sum of two angles is 139° and their difference is 5°; find each angle of the triangle.
उत्तर
Let ABC be a triangle such that
∠A + ∠B = 139° ....(i)
and ∠A - ∠B = 5° ....(ii)
Adding (i) and (ii), we get
2∠A = 144°
⇒ ∠A = 72°
From (i), we have
∠B = 139° - 72° = 67°
Now, 3rd angle
= 180° - (∠A + ∠B)
= 180° - 139°
= 41°
Thus, the angles of a triangle are 72°, 67° and 41°.
APPEARS IN
संबंधित प्रश्न
In the given figure, ∠Q: ∠R = 1: 2. Find:
a. ∠Q
b. ∠R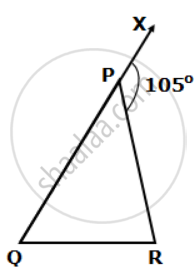
The exterior angles, obtained on producing the side of a triangle both ways, are 100° and 120°. Find all the angles of the triangle.
Use the given figure to find the value of x in terms of y. Calculate x, if y = 15°.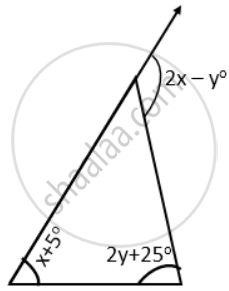
In a triangle PQR, ∠P + ∠Q = 130° and ∠P + ∠R = 120°. Calculate each angle of the triangle.
Use the given figure to find the value of y in terms of p, q and r.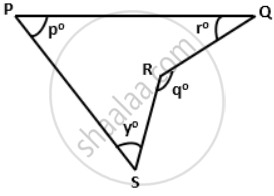
In the figure given below, if RS is parallel to PQ, then find the value of ∠y.
Use the given figure to show that: ∠p + ∠q + ∠r = 360°.
In a triangle ABC, if the bisectors of angles ABC and ACB meet at M then prove that: ∠BMC = 90° + `(1)/(2)` ∠A.
If bisectors of angles A and D of a quadrilateral ABCD meet at 0, then show that ∠B + ∠C = 2 ∠AOD
In a right-angled triangle ABC, ∠B = 90°. If BA and BC produced to the points P and Q respectively, find the value of ∠PAC + ∠QCA.
