Advertisements
Chapters
2: Profit , Loss and Discount
3: Compound Interest
4: Expansions
5: Factorisation
6: Changing the subject of a formula
7: Linear Equations
8: Simultaneous Linear Equations
9: Indices
10: Logarithms
▶ 11: Triangles and their congruency
12: Isosceles Triangle
13: Inequalities in Triangles
14: Constructions of Triangles
15: Mid-point and Intercept Theorems
16: Similarity
17: Pythagoras Theorem
18: Rectilinear Figures
19: Quadrilaterals
20: Constructions of Quadrilaterals
21: Areas Theorems on Parallelograms
22: Statistics
23: Graphical Representation of Statistical Data
24: Perimeter and Area
25: Surface Areas and Volume of Solids
26: Trigonometrical Ratios
27: Trigonometrical Ratios of Standard Angles
28: Coordinate Geometry
![Frank solutions for Mathematics [English] Class 9 ICSE chapter 11 - Triangles and their congruency Frank solutions for Mathematics [English] Class 9 ICSE chapter 11 - Triangles and their congruency - Shaalaa.com](/images/mathematics-english-class-9-icse_6:c41cc344f5174c64a036c55d113af73f.jpg)
Advertisements
Solutions for Chapter 11: Triangles and their congruency
Below listed, you can find solutions for Chapter 11 of CISCE Frank for Mathematics [English] Class 9 ICSE.
Frank solutions for Mathematics [English] Class 9 ICSE 11 Triangles and their congruency Exercise 11.1
In the given figure, ∠Q: ∠R = 1: 2. Find:
a. ∠Q
b. ∠R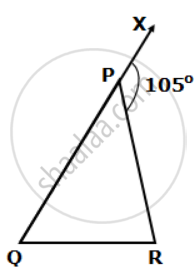
The exterior angles, obtained on producing the side of a triangle both ways, are 100° and 120°. Find all the angles of the triangle.
Use the given figure to find the value of x in terms of y. Calculate x, if y = 15°.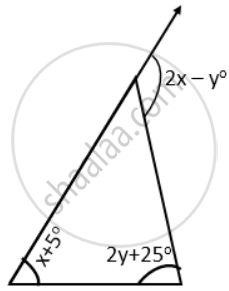
In a triangle PQR, ∠P + ∠Q = 130° and ∠P + ∠R = 120°. Calculate each angle of the triangle.
The angles of a triangle are (x + 10)°, (x + 30)° and (x - 10)°. Find the value of 'x'. Also, find the measure of each angle of the triangle.
Use the given figure to find the value of y in terms of p, q and r.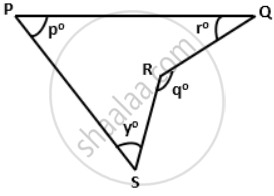
In the figure given below, if RS is parallel to PQ, then find the value of ∠y.
In a triangle PQR, the internal bisectors of angles Q and R meet at A and the external bisectors of the angles Q and R meet at B. Prove that: ∠QAR + ∠QBR = 180°.
Use the given figure to show that: ∠p + ∠q + ∠r = 360°.
In a triangle ABC. If D is a point on BC such that ∠CAD = ∠B, then prove that: ∠ADC = ∠BAC.
In a triangle ABC, if the bisectors of angles ABC and ACB meet at M then prove that: ∠BMC = 90° + `(1)/(2)` ∠A.
If bisectors of angles A and D of a quadrilateral ABCD meet at 0, then show that ∠B + ∠C = 2 ∠AOD
If each angle of a triangle is less than the sum of the other two angles of it; prove that the triangle is acute-angled.
If the angles of a triangle are in the ratio 2: 4: 6; show that the triangle is a right-angled triangle.
In a triangle, the sum of two angles is 139° and their difference is 5°; find each angle of the triangle.
In a right-angled triangle ABC, ∠B = 90°. If BA and BC produced to the points P and Q respectively, find the value of ∠PAC + ∠QCA.
Frank solutions for Mathematics [English] Class 9 ICSE 11 Triangles and their congruency Exercise 11.2
Which of the following pairs of triangles are congruent? Give reasons
ΔABC;(BC = 5cm,AC = 6cm,∠C = 80°);
ΔXYZ;(XZ = 6cm,XY = 5cm,∠X = 70°).
Which of the following pairs of triangles are congruent? Give reasons
ΔABC;(AB = 8cm,BC = 6cm,∠B = 100°);
ΔPQR;(PQ = 8cm,RP = 5cm,∠Q = 100°).
Which of the following pairs of triangles are congruent? Give reasons
ΔABC;(AB = 5cm,BC = 7cm,CA = 9cm);
ΔKLM;(KL = 7cm,LM = 5cm,KM = 9cm).
Which of the following pairs of triangles are congruent? Give reasons
ΔABC;(∠B = 70°,BC = 6cm,∠C = 50°);
ΔXYZ;(∠Z = 60°,XY = 6cm,∠X = 70°).
Which of the following pairs of triangles are congruent? Give reasons
ΔABC;(∠B = 90°,BC = 6cm,AB = 8cm);
ΔPQR;(∠Q = 90°,PQ = 6cm,PR = 10cm).
A is any point in the angle PQR such that the perpendiculars drawn from A on PQ and QR are equal. Prove that ∠AQP = ∠AQR.
In the given figure P is a midpoint of chord AB of the circle O. prove that OP ^ AB.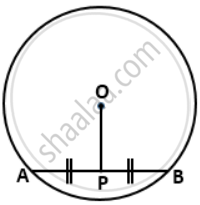
In a circle with center O. If OM is perpendicular to PQ, prove that PM = QM.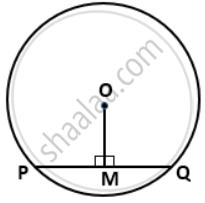
In ΔABC and ΔPQR and, AB = PQ, BC = QR and CB and RQ are extended to X and Y respectively and ∠ABX = ∠PQY. = Prove that ΔABC ≅ ΔPQR.
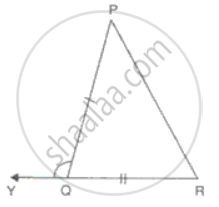
In a triangle ABC, if D is midpoint of BC; AD is produced upto E such as DE = AD, then prove that:
a. DABD andDECD are congruent.
b. AB = EC
c. AB is parallel to EC
In the figure, ∠CPD = ∠BPD and AD is the bisector of ∠BAC. Prove that ΔCAP ≅ ΔBAP and CP = BP.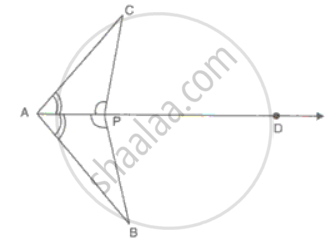
In the figure, BC = CE and ∠1 = ∠2. Prove that ΔGCB ≅ ΔDCE.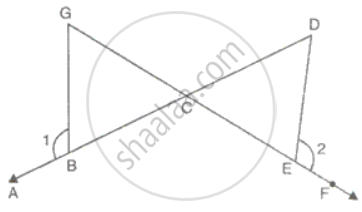
In ΔABC, AB = AC and the bisectors of angles B and C intersect at point O.Prove that BO = CO and the ray AO is the bisector of angle BAC.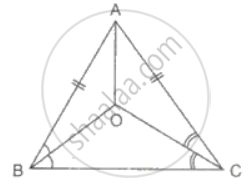
In the figure, AB = EF, BC = DE, AB and FE are perpendiculars on BE. Prove that ΔABD ≅ ΔFEC
In the figure, BM and DN are both perpendiculars on AC and BM = DN. Prove that AC bisects BD.
In ΔPQR, LM = MN, QM = MR and ML and MN are perpendiculars on PQ and PR respectively. Prove that PQ = PR.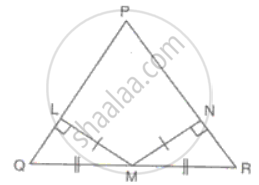
In the figure, RT = TS, ∠1 = 2∠2 and ∠4 = 2∠3. Prove that ΔRBT ≅ ΔSAT.
AD and BE are altitudes of an isosceles triangle ABC with AC = BC. Prove that AE = BD.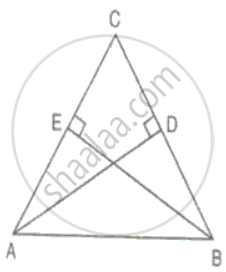
In ΔABC, X and Y are two points on AB and AC such that AX = AY. If AB = AC, prove that CX = BY.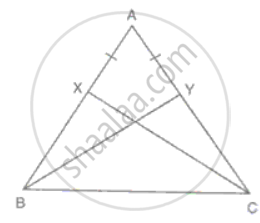
If the perpendicular bisector of the sides of a triangle PQR meet at I, then prove that the line joining from P, Q, R to I are equal.
In the figure, AC = AE, AB = AD and ∠BAD = ∠EAC. Prove that BC = DE.
In the given figure ABCD is a parallelogram, AB is Produced to L and E is a midpoint of BC. Show that:
a. DDCE ≅ DLDE
b. AB = BL
c. DC = `"AL"/(2)`
In the figure, ∠BCD = ∠ADC and ∠ACB =∠BDA. Prove that AD = BC and ∠A = ∠B.
In the figure, AP and BQ are perpendiculars to the line segment AB and AP = BQ. Prove that O is the mid-point of the line segments AB and PQ.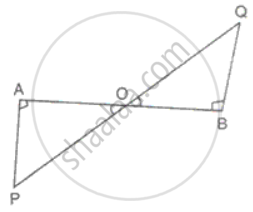
ΔABC is isosceles with AB = AC. BD and CE are two medians of the triangle. Prove that BD = CE.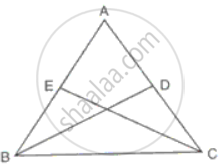
Sides, AB, BC and the median AD of ΔABC are equal to the two sides PQ, QR and the median PM of ΔPQR. Prove that ΔABC ≅ ΔPQR.
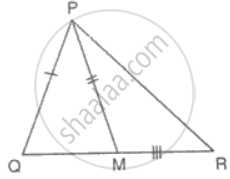
Prove that in an isosceles triangle the altitude from the vertex will bisect the base.
In ΔABC, AB = AC. D is a point in the interior of the triangle such that ∠DBC = ∠DCB. Prove that AD bisects ∠BAC of ΔABC.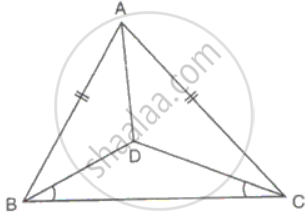
O is any point in the ΔABC such that the perpendicular drawn from O on AB and AC are equal. Prove that OA is the bisector of ∠BAC.
In ΔABC, AB = AC, BM and Cn are perpendiculars on AC and AB respectively. Prove that BM = CN.
ΔABC is an isosceles triangle with AB = AC. GB and HC ARE perpendiculars drawn on BC.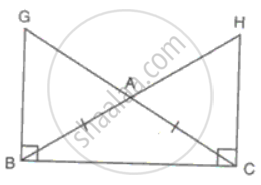
Prove that
(i) BG = CH
(ii) AG = AH
In ΔABC, AD is a median. The perpendiculars from B and C meet the line AD produced at X and Y. Prove that BX = CY.
Two right-angled triangles ABC and ADC have the same base AC. If BC = DC, prove that AC bisects ∠BCD.
PQRS is a quadrilateral and T and U are points on PS and RS respectively such that PQ = RQ, ∠PQT = ∠RQU and ∠TQS = ∠UQS. Prove that QT = QU.
In the given figure, AB = DB and AC = DC. Find the values of x and y.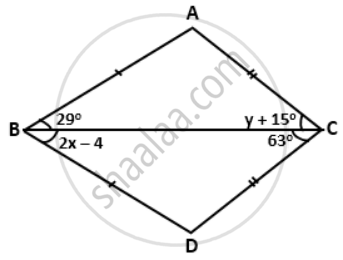
Solutions for 11: Triangles and their congruency
![Frank solutions for Mathematics [English] Class 9 ICSE chapter 11 - Triangles and their congruency Frank solutions for Mathematics [English] Class 9 ICSE chapter 11 - Triangles and their congruency - Shaalaa.com](/images/mathematics-english-class-9-icse_6:c41cc344f5174c64a036c55d113af73f.jpg)
Frank solutions for Mathematics [English] Class 9 ICSE chapter 11 - Triangles and their congruency
Shaalaa.com has the CISCE Mathematics Mathematics [English] Class 9 ICSE CISCE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. Frank solutions for Mathematics Mathematics [English] Class 9 ICSE CISCE 11 (Triangles and their congruency) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. Frank textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 9 ICSE chapter 11 Triangles and their congruency are Relation Between Sides and Angles of Triangle, Important Terms of Triangle, Congruence of Triangles, Criteria for Congruence of Triangles, Concept of Triangles.
Using Frank Mathematics [English] Class 9 ICSE solutions Triangles and their congruency exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in Frank Solutions are essential questions that can be asked in the final exam. Maximum CISCE Mathematics [English] Class 9 ICSE students prefer Frank Textbook Solutions to score more in exams.
Get the free view of Chapter 11, Triangles and their congruency Mathematics [English] Class 9 ICSE additional questions for Mathematics Mathematics [English] Class 9 ICSE CISCE, and you can use Shaalaa.com to keep it handy for your exam preparation.
