Advertisements
Advertisements
प्रश्न
ΔABC is isosceles with AB = AC. BD and CE are two medians of the triangle. Prove that BD = CE.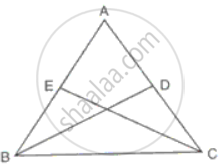
उत्तर
CE is median to AB
⇒ AE = BE ......(i)
BD is median to AC
⇒ AD = DC ......(i)
But AB =AC ......(iii)
Therefore from (i), (ii) and (iii)
BE = CD
In ΔBEC and ΔBDC
BE = CD
∠EBC = ∠DCB ...(angles opposites to equal sides are equal)
BC = BC ...(common)
Therefore, ΔBEC ≅ ΔBDC ...(SAS criteria)
Hence, BD = CE.
APPEARS IN
संबंधित प्रश्न
If ΔABC ≅ ΔFED under the correspondence ABC ↔ FED, write all the Corresponding congruent parts of the triangles.
Find the measure of each angle of an equilateral triangle.
ΔPQR and ΔABC is not congruent to ΔRPQ, then which of the following is not true:
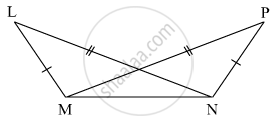
As shown in the following figure, in ΔLMN and ΔPNM, LM = PN, LN = PM. Write the test which assures the congruence of the two triangles. Write their remaining congruent parts.
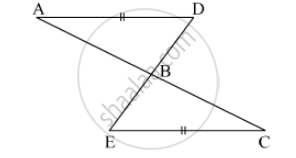
In the adjacent figure, seg AD ≌ seg EC Which additional information is needed to show that ∆ ABD and ∆ EBC will be congruent by A-A-S test?
The following figure has shown a triangle ABC in which AB = AC. M is a point on AB and N is a point on AC such that BM = CN.
Prove that: (i) BN = CM (ii) ΔBMC≅ΔCNB

State, whether the pairs of triangles given in the following figures are congruent or not:


Which of the following pairs of triangles are congruent? Give reasons
ΔABC;(∠B = 70°,BC = 6cm,∠C = 50°);
ΔXYZ;(∠Z = 60°,XY = 6cm,∠X = 70°).
O is any point in the ΔABC such that the perpendicular drawn from O on AB and AC are equal. Prove that OA is the bisector of ∠BAC.
In triangles ABC and DEF, AB = FD and ∠A = ∠D. The two triangles will be congruent by SAS axiom if ______.
