Advertisements
Chapters
2: Profit , Loss and Discount
3: Compound Interest
4: Expansions
5: Factorisation
6: Changing the subject of a formula
7: Linear Equations
8: Simultaneous Linear Equations
9: Indices
10: Logarithms
11: Triangles and their congruency
12: Isosceles Triangle
13: Inequalities in Triangles
14: Constructions of Triangles
15: Mid-point and Intercept Theorems
16: Similarity
17: Pythagoras Theorem
18: Rectilinear Figures
19: Quadrilaterals
20: Constructions of Quadrilaterals
21: Areas Theorems on Parallelograms
22: Statistics
23: Graphical Representation of Statistical Data
24: Perimeter and Area
25: Surface Areas and Volume of Solids
26: Trigonometrical Ratios
▶ 27: Trigonometrical Ratios of Standard Angles
28: Coordinate Geometry
![Frank solutions for Mathematics [English] Class 9 ICSE chapter 27 - Trigonometrical Ratios of Standard Angles Frank solutions for Mathematics [English] Class 9 ICSE chapter 27 - Trigonometrical Ratios of Standard Angles - Shaalaa.com](/images/mathematics-english-class-9-icse_6:c41cc344f5174c64a036c55d113af73f.jpg)
Advertisements
Solutions for Chapter 27: Trigonometrical Ratios of Standard Angles
Below listed, you can find solutions for Chapter 27 of CISCE Frank for Mathematics [English] Class 9 ICSE.
Frank solutions for Mathematics [English] Class 9 ICSE 27 Trigonometrical Ratios of Standard Angles Exercise 27.1
Without using tables, evaluate the following: sin60° sin30°+ cos30° cos60°
Without using tables, evaluate the following: sec30° cosec60° + cos60° sin30°.
Without using tables, evaluate the following sec45° sin45° - sin30° sec60°.
Without using tables, evaluate the following: sin230° sin245° + sin260° sin290°.
Without using tables, evaluate the following: tan230° + tan260° + tan245°
Without using tables, evaluate the following: sin230° cos245° + 4tan230° + sin290° + cos20°
Without using tables, evaluate the following: cosec245° sec230° - sin230° - 4cot245° + sec260°.
Without using tables, evaluate the following: cosec330° cos60° tan345° sin290° sec245° cot30°.
Without using tables, evaluate the following: (sin90° + sin45° + sin30°)(sin90° - cos45° + cos60°).
Without using tables, evaluate the following: 4(sin430° + cos460°) - 3(cos245° - sin290°).
Without using table, find the value of the following:
Without using tables, find the value of the following:
Without using tables, find the value of the following:
Without using table, find the value of the following:
Without using tables, find the value of the following:
Prove that: sin60°. cos30° - sin60°. sin30° =
Prove that : cos60° . cos30° - sin60° . sin30° = 0
Prove that : sec245° - tan245° = 1
Prove that:
Find the value of 'A', if 2 cos A = 1
Find the value of 'A', if 2 sin 2A = 1
Find the value of 'A', if cosec 3A =
Find the value of 'A', if 2cos 3A = 1
Find the value of 'A', if
Find the value of 'A', if cot 3A = 1
Find the value of 'A', if (1 - cosec A)(2 - sec A) = 0
Find the value of 'A', if (2 - cosec 2A) cos 3A = 0
If sin α + cosβ = 1 and α= 90°, find the value of 'β'.
Solve for 'θ':
Solve for 'θ': cot2(θ - 5)° = 3
Solve for 'θ':
Find the value of x in the following: 2 sin3x =
Find the value of x in the following:
Find the value of x in the following:
Find the value of x in the following: tan x = sin45° cos45° + sin30°
Find the value of x in the following:
Find the value of x in the following: cos2x = cos60° cos30° + sin60° sin30°
If sinθ = cosθ and 0° < θ<90°, find the value of 'θ'.
If tanθ= cotθ and 0°≤ θ ≤ 90°, find the value of 'θ'.
If
If θ = 30°, verify that: tan2θ =
If θ = 30°, verify that: sin2θ =
If A = 30°, verify that cos2θ =
If θ = 30°, verify that: sin 3θ = 4sinθ . sin(60° - θ) sin(60° + θ)
If θ = 30°, verify that: 1 - sin 2θ = (sinθ - cosθ)2
Evaluate the following:
Evaluate the following:
If θ = 15°, find the value of: cos3θ - sin6θ + 3sin(5θ + 15°) - 2 tan23θ
If A = B = 60°, verify that: cos(A - B) = cosA cosB + sinA sinB
If A = B = 60°, verify that: sin(A - B) = sinA cosB - cosA sinB
If A = B = 60°, verify that: tan(A - B) =
If A = 30° and B = 60°, verify that: sin (A + B) = sin A cos B + cos A sin B
If A = 30° and B = 60°, verify that: cos (A + B) = cos A cos B - sin A sin B
If A = 30° and B = 60°, verify that:
If A = 30° and B = 60°, verify that:
If A = B = 45°, verify that sin (A - B) = sin A .cos B - cos A.sin B
If A = B = 45°, verify that cos (A − B) = cos A. cos B + sin A. sin B
If sin(A - B) = sinA cosB - cosA sinB and cos(A - B) = cosA cosB + sinA sinB, find the values of sin15° and cos15°.
If θ < 90°, find the value of: sin2θ + cos2θ
If θ < 90°, find the value of:
If
If
cos 3θ
If
cos2 (30° + θ) + sin2 (45° - θ)
In the given figure, PQ = 6 cm, RQ = x cm and RP = 10 cm, find
a. cosθ
b. sin2θ- cos2θ
c. Use tanθ to find the value of RQ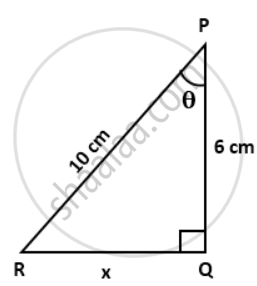
Find the value of:
a. cosθ
b. sinθ
If sin(A +B) = 1(A -B) = 1, find A and B.
If tan(A - B) =
If sin(A - B) =
In ΔABC right angled at B, ∠A = ∠C. Find the value of:
(i) sinA cosC + cosA sinC
(ii) sinA sinB + cosA cosB
If tan
Frank solutions for Mathematics [English] Class 9 ICSE 27 Trigonometrical Ratios of Standard Angles Exercise 27.2
Find the value of 'x' in each of the following: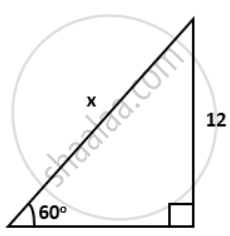
Find the value of 'x' in each of the following: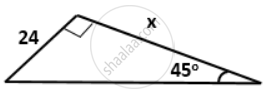
Find the value of 'x' in each of the following: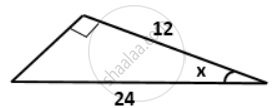
Find the value of 'x' in each of the following:
Find the length of AD. Given: ∠ABC = 60°, ∠DBC = 45° and BC = 24 cm.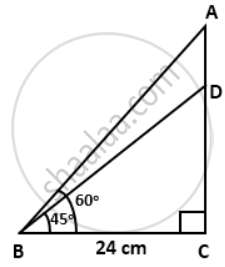
Find lengths of diagonals AC and BD. Given AB = 24 cm and ∠BAD = 60°.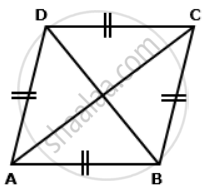
In a trapezium ABCD, as shown, AB ‖ DC, AD = DC = BC = 24 cm and ∠A = 30°. Find: length of AB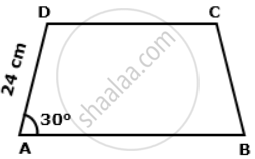
Find the length of EC.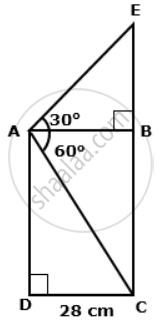
In the given figure, AB and EC are parallel to each other. Sides AD and BC are 1.5 cm each and are perpendicular to AB. Given that ∠AED = 45° and ∠ACD = 30°. Find:
a. AB
b. AC
c. AE
In the given figure, ∠B = 60°, ∠C = 30°, AB = 8 cm and BC = 24 cm. Find:
a. BE
b. AC
Find:
a. BC
b. AD
c. AC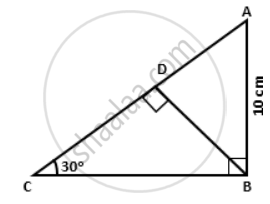
Find the value 'x', if:
Find the value 'x', if: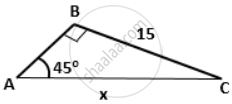
Find the value 'x', if: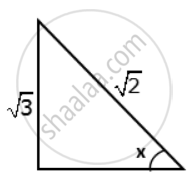
Find the value 'x', if: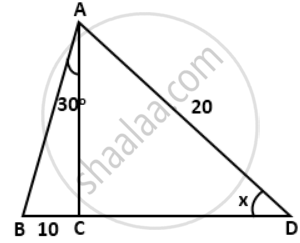
Find the value 'x', if: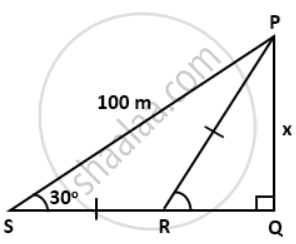
Find the value 'x', if:
Find the value of 'y' if
Given your answer correct to 2 decimal places.
Find the value of 'y' if
Given your answer correct to 2 decimal places.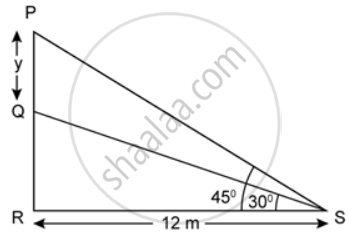
In the given figure, if tan θ = 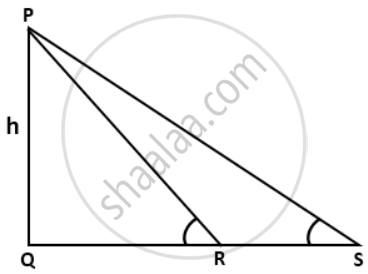
Find x and y, in each of the following figure: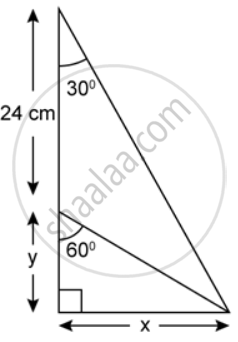
Find x and y, in each of the following figure: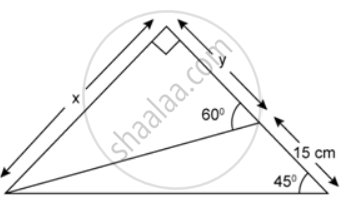
If tan x° = 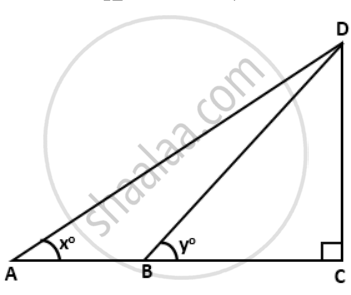
In a right triangle ABC, right angled at C, if ∠B = 60° and AB = 15units, find the remaining angles and sides.
If ΔABC is a right triangle such that ∠C = 90°, ∠A = 45° and BC =7units, find ∠B, AB and AC.
In a rectangle ABCD, AB = 20cm, ∠BAC = 60°, calculate side BC and diagonals AC and BD.
In right-angled triangle ABC; ∠B = 90°. Find the magnitude of angle A, if:
a. AB is
B. BC is
A ladder is placed against a vertical tower. If the ladder makes an angle of 30° with the ground and reaches upto a height of 18 m of the tower; find length of the ladder.
The perimeter of a rhombus is 100 cm and obtuse angle of it is 120°. Find the lengths of its diagonals.
In the given figure; ∠B = 90°, ∠ADB = 30°, ∠ACB = 45° and AB = 24 m. Find the length of CD.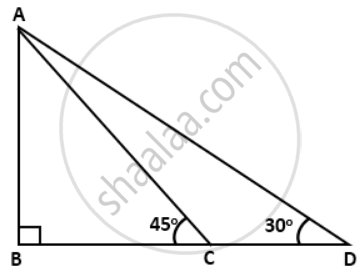
In the given figure, a rocket is fired vertically upwards from its launching pad P. It first rises 20 km vertically upwards and then 20 km at 60° to the vertical. PQ represents the first stage of the journey and QR the second. S is a point vertically below R on the horizontal level as P, find:
a. the height of the rocket when it is at point R.
b. the horizontal distance of point S from P.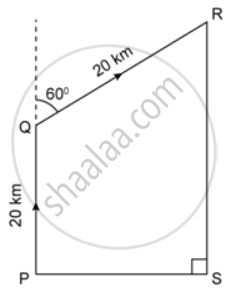
Frank solutions for Mathematics [English] Class 9 ICSE 27 Trigonometrical Ratios of Standard Angles Exercise 27.3
Evaluate the following:
Evaluate the following:
Evaluate the following:
Evaluate the following:
Evaluate the following:
Evaluate the following:
Evaluate the following: sin31° - cos59°
Evaluate the following: cot27° - tan63°
Evaluate the following: cosec 54° - sec 36°
Evaluate the following: sin28° sec62° + tan49° tan41°
Evaluate the following: sec16° tan28° - cot62° cosec74°
Evaluate the following: sin22° cos44° - sin46° cos68°
Evaluate the following:
Evaluate the following:
Evaluate the following:
Evaluate the following:
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: sin65° + cot59°
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: cos72° - cos88°
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: cosec64° + sec70°
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: tan77° - cot63° + sin57°
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: sin53° + sec66° - sin50°
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: cos84° + cosec69° - cot68°
Evaluate the following: sin35° sin45° sec55° sec45°
Evaluate the following: cot20° cot40° cot45° cot50° cot70°
Evaluate the following: cos39° cos48° cos60° cosec42° cosec51°
Evaluate the following: sin(35° + θ) - cos(55° - θ) - tan(42° + θ) + cot(48° - θ)
Evaluate the following: tan(78° + θ) + cosec(42° + θ) - cot(12° - θ) - sec(48° - θ)
Evaluate the following:
Evaluate the following:
Evaluate the following:
Evaluate the following:
Evaluate the following:
If cos3θ = sin(θ - 34°), find the value of θ if 3θ is an acute angle.
If tan4θ = cot(θ + 20°), find the value of θ if 4θ is an acute angle.
If sec2θ = cosec3θ, find the value of θ if it is known that both 2θ and 3θ are acute angles.
If sin(θ - 15°) = cos(θ - 25°), find the value of θ if (θ-15°) and (θ - 25°) are acute angles.
If A, B and C are interior angles of ΔABC, prove that sin
If P, Q and R are the interior angles of ΔPQR, prove that
If cosθ = sin60° and θ is an acute angle find the value of 1- 2 sin2θ
If secθ= cosec30° and θ is an acute angle, find the value of 4 sin2θ - 2 cos2θ.
Prove the following: tanθ tan(90° - θ) = cotθ cot(90° - θ)
Prove the following: sin58° sec32° + cos58° cosec32° = 2
Prove the following:
Prove the following: sin230° + cos230° =
If A + B = 90°, prove that
Solutions for 27: Trigonometrical Ratios of Standard Angles
![Frank solutions for Mathematics [English] Class 9 ICSE chapter 27 - Trigonometrical Ratios of Standard Angles Frank solutions for Mathematics [English] Class 9 ICSE chapter 27 - Trigonometrical Ratios of Standard Angles - Shaalaa.com](/images/mathematics-english-class-9-icse_6:c41cc344f5174c64a036c55d113af73f.jpg)
Frank solutions for Mathematics [English] Class 9 ICSE chapter 27 - Trigonometrical Ratios of Standard Angles
Shaalaa.com has the CISCE Mathematics Mathematics [English] Class 9 ICSE CISCE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. Frank solutions for Mathematics Mathematics [English] Class 9 ICSE CISCE 27 (Trigonometrical Ratios of Standard Angles) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. Frank textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 9 ICSE chapter 27 Trigonometrical Ratios of Standard Angles are Trigonometric Equation Problem and Solution, Trigonometric Ratios of Some Special Angles, Trigonometric Ratios of Some Special Angles, Trigonometric Ratios of Some Special Angles.
Using Frank Mathematics [English] Class 9 ICSE solutions Trigonometrical Ratios of Standard Angles exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in Frank Solutions are essential questions that can be asked in the final exam. Maximum CISCE Mathematics [English] Class 9 ICSE students prefer Frank Textbook Solutions to score more in exams.
Get the free view of Chapter 27, Trigonometrical Ratios of Standard Angles Mathematics [English] Class 9 ICSE additional questions for Mathematics Mathematics [English] Class 9 ICSE CISCE, and you can use Shaalaa.com to keep it handy for your exam preparation.
