Question

The perimeter of a rhombus is 100 cm and obtuse angle of it is 120°. Find the lengths of its diagonals.

Answer 1

Consider the following figure,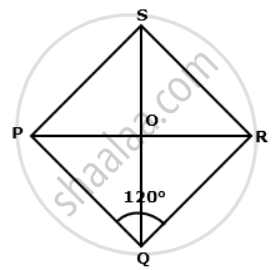

Perimeter of rhombus = 100cm

⇒ PQ = QR = RS = SP = `(100)/(4)` = 25cm

Diagonals of a rhombus bisect each other ar right angles.
⇒ PO = OR and QO = OS
And,
∠POQ = ∠ROQ = ∠ROS = ∠POS = 90°
Also, diagonals bisect the angle at vertex.

⇒ `∠"PQO" = (1)/(2) ∠"POQ" = (1)/(2) xx 120° = 60°`

Now, In right ΔPQR,

sin(∠PQO) = `"OP"/"PQ"`

⇒ sin60° = `"OP"/(25)`

⇒ `sqrt(3)/(2) = "OP"/(25)`

⇒ OP = `(25sqrt(3))/(2)`

∴ PR
= 2 x OP

= `2 xx (25sqrt(3))/(2)`
= `25sqrt(3)"cm"`

Also,
cos(∠PQO) = `"OQ"/"PQ"`

⇒ cos60 = `"OQ"/(25)`

⇒ `(1)/(2) = "OQ"/(25)`

⇒ OQ = `(25)/(2)`

∴ SQ
= 2 x OQ

= `2 xx (25)/(2)`
= 25cm.