Advertisements
Advertisements
प्रश्न
Evaluate the following: `(sin36°)/(cos54°) + (sec31°)/("cosec"59°)`
उत्तर
`(sin36°)/(cos54°) + (sec31°)/("cosec"59°)`
= `(sin(90° - 54°))/(cos54°) + (sec(90° - 59°))/("cosec"59°)`
= `(cos54°)/(cos54°) + ("cosec"59°)/("cosec"59°)`
= 1 + 1
= 2.
APPEARS IN
संबंधित प्रश्न
If 3 tan A - 5 cos B = `sqrt3` and B = 90°, find the value of A
Solve for x : cos2 30° + sin2 2x = 1
Find the value of 'A', if (2 - cosec 2A) cos 3A = 0
If tanθ= cotθ and 0°≤ θ ≤ 90°, find the value of 'θ'.
If θ = 30°, verify that: tan2θ = `(2tanθ)/(1 - tan^2θ)`
Find the value 'x', if: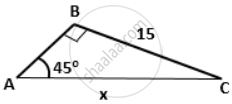
In the given figure, if tan θ = `(5)/(13), tan α = (3)/(5)` and RS = 12m, find the value of 'h'.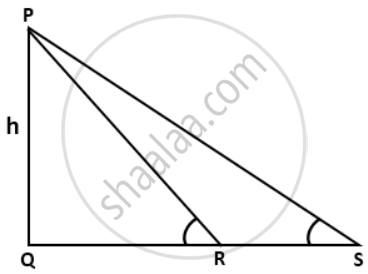
Evaluate the following: cos39° cos48° cos60° cosec42° cosec51°
Evaluate the following: `(3sin^2 40°)/(4cos^2 50°) - ("cosec"^2 28°)/(4sec^2 62°) + (cos10° cos25° cos45° "cosec"80°)/(2sin15° sin25° sin45° sin65° sec75°)`
If sec2θ = cosec3θ, find the value of θ if it is known that both 2θ and 3θ are acute angles.
