Advertisements
Advertisements
प्रश्न
If θ = 30°, verify that: 1 - sin 2θ = (sinθ - cosθ)2
योग
उत्तर
Given: θ = 30°
1 - sin2θ
= 1 - sin2 x 30°
= 1 - sin60°
=
=
(sinθ - cosθ)2
= sin2θ + cos2θ - 2sinθ cosθ
= 1 - 2 x sin30° x cos30
=
=
=
⇒ 1 - sin2θ = (sinθ - cosθ)2.
shaalaa.com
Trigonometric Equation Problem and Solution
क्या इस प्रश्न या उत्तर में कोई त्रुटि है?
APPEARS IN
संबंधित प्रश्न
Solve for x : cos
Solve for x : 3 tan2 (2x - 20°) = 1
Find the value of 'A', if (1 - cosec A)(2 - sec A) = 0
If θ = 30°, verify that: sin 3θ = 4sinθ . sin(60° - θ) sin(60° + θ)
If θ = 15°, find the value of: cos3θ - sin6θ + 3sin(5θ + 15°) - 2 tan23θ
Find:
a. BC
b. AD
c. AC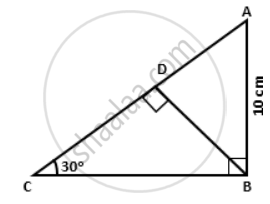
Evaluate the following:
Evaluate the following: sec16° tan28° - cot62° cosec74°
Evaluate the following: cos39° cos48° cos60° cosec42° cosec51°
If cosθ = sin60° and θ is an acute angle find the value of 1- 2 sin2θ
