Advertisements
Advertisements
प्रश्न
If sin(θ - 15°) = cos(θ - 25°), find the value of θ if (θ-15°) and (θ - 25°) are acute angles.
उत्तर
sin(θ - 15°) = cos(θ - 25°)
⇒ cos[90° - (θ - 15°)] = cos(θ - 25°)
⇒ 90° - (θ - 15°) = θ - 25°
⇒ 105° - θ = θ - 25°
⇒ 2θ = 130°
⇒ θ = 65°.
APPEARS IN
संबंधित प्रश्न
Calculate the value of A, if (sec 2A - 1) (cosec 3A - 1) = 0
Solve for x : sin2 x + sin2 30° = 1
Find the value of 'A', if `sqrt(3)cot"A"` = 1
Find the length of EC.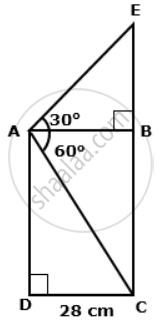
Find the value 'x', if: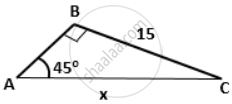
A ladder is placed against a vertical tower. If the ladder makes an angle of 30° with the ground and reaches upto a height of 18 m of the tower; find length of the ladder.
Evaluate the following: sin28° sec62° + tan49° tan41°
Evaluate the following: `(3sin37°)/(cos53°) - (5"cosec"39°)/(sec51°) + (4tan23° tan37° tan67° tan53°)/(cos17° cos67° "cosec"73° "cosec"23°)`
If A, B and C are interior angles of ΔABC, prove that sin`(("A" + "B")/2) = cos "C"/(2)`
Prove the following: `(tan(90° - θ)cotθ)/("cosec"^2 θ)` = cos2θ
