Advertisements
Advertisements
प्रश्न
If sin(θ - 15°) = cos(θ - 25°), find the value of θ if (θ-15°) and (θ - 25°) are acute angles.
उत्तर
sin(θ - 15°) = cos(θ - 25°)
⇒ cos[90° - (θ - 15°)] = cos(θ - 25°)
⇒ 90° - (θ - 15°) = θ - 25°
⇒ 105° - θ = θ - 25°
⇒ 2θ = 130°
⇒ θ = 65°.
APPEARS IN
संबंधित प्रश्न
In ΔABC, ∠B = 90° , AB = y units, BC = `(sqrt3)` units, AC = 2 units and angle A = x°, find:
- sin x°
- x°
- tan x°
- use cos x° to find the value of y.
State for any acute angle θ whether cos θ increases or decreases as θ increases.
Solve the following equation for A, if 2 sin 3 A = 1
Find the value of 'A', if 2 cos A = 1
Solve for 'θ': cot2(θ - 5)° = 3
If `sqrt(2) = 1.414 and sqrt(3) = 1.732`, find the value of the following correct to two decimal places tan60°
In the given figure, if tan θ = `(5)/(13), tan α = (3)/(5)` and RS = 12m, find the value of 'h'.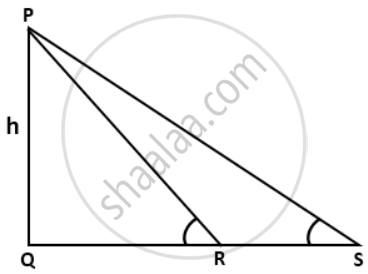
Evaluate the following: cot27° - tan63°
Evaluate the following: `(5sec68°)/("cosec"22°) + (3sin52° sec38°)/(cot51° cot39°)`
If A + B = 90°, prove that `(tan"A" tan"B" + tan"A" cot"B")/(sin"A" sec"B") - (sin^2"B")/(cos^2"A")` = tan2A
