Advertisements
Advertisements
प्रश्न
Evaluate the following: `(3sin37°)/(cos53°) - (5"cosec"39°)/(sec51°) + (4tan23° tan37° tan67° tan53°)/(cos17° cos67° "cosec"73° "cosec"23°)`
उत्तर
`(3sin37°)/(cos53°) - (5"cosec"39°)/(sec51°) + (4tan23° tan37° tan67° tan53°)/(cos17° cos67° "cosec"73° "cosec"23°)`
= `(3sin(90° - 53°))/(cos53°) - (5"cosec"(90° - 51°))/(sec51°) + (4tan(90° - 67°) tan(90° - 53°) xx 1/(cot67°) xx 1/(cot53°))/(cos(90° - 73°) cos(90° - 23°) xx 1/(sin73°) xx 1/(sin23°)`
= `(3cos53°)/(cos53°) - (5sec51°)/(sec51°) + (4 cos67° cos53° xx 1/(cot67°) xx 1/cot53°)/(sin73° sin23° xx 1/(sin73°) xx 1/sin23°)`
= 3 - 5 + 4
= 2.
APPEARS IN
संबंधित प्रश्न
From the given figure,
find:
(i) cos x°
(ii) x°
(iii) `(1)/(tan^2 xx°) – (1)/(sin^2xx°)`
(iv) Use tan xo, to find the value of y.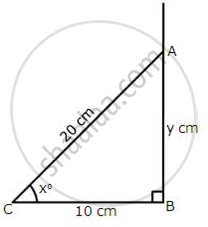
Solve for x : 2 cos 3x - 1 = 0
Calculate the value of A, if (cosec 2A - 2) (cot 3A - 1) = 0
Solve for x : sin2 x + sin2 30° = 1
Find the value of: `sqrt((1 - sin^2 60°)/(1 + sin^2 60°)` If 3 tan2θ - 1 = 0, find the value
a. cosθ
b. sinθ
Find:
a. BC
b. AD
c. AC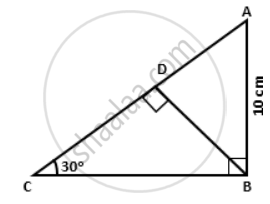
The perimeter of a rhombus is 100 cm and obtuse angle of it is 120°. Find the lengths of its diagonals.
Evaluate the following: `(sec34°)/("cosec"56°)`
Evaluate the following: sin31° - cos59°
Prove the following: `(tan(90° - θ)cotθ)/("cosec"^2 θ)` = cos2θ
