Advertisements
Advertisements
प्रश्न
Solve for x : sin2 x + sin2 30° = 1
उत्तर
sin2x + sin230° = 1
sin2x = 1 –sin2 30°
sin2x = 1 – `(1)/(4)`
sin2x = `(sqrt3)/(2)`
x = 60°
APPEARS IN
संबंधित प्रश्न
If 4 sin2 θ - 1= 0 and angle θ is less than 90°, find the value of θ and hence the value of cos2 θ + tan2θ.
If 2 cos 2A = `sqrt3` and A is acute,
find:
(i) A
(ii) sin 3A
(iii) sin2 (75° - A) + cos2 (45° +A)
If `sqrt(2) = 1.414 and sqrt(3) = 1.732`, find the value of the following correct to two decimal places tan60°
Evaluate the following: `((sin3θ - 2sin4θ))/((cos3θ - 2cos4θ))` when 2θ = 30°
If A = B = 60°, verify that: cos(A - B) = cosA cosB + sinA sinB
Find the value of: `sqrt((1 - sin^2 60°)/(1 + sin^2 60°)` If 3 tan2θ - 1 = 0, find the value
a. cosθ
b. sinθ
Find the value of 'x' in each of the following: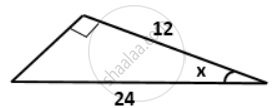
Find the value of 'y' if `sqrt(3)` = 1.723.
Given your answer correct to 2 decimal places.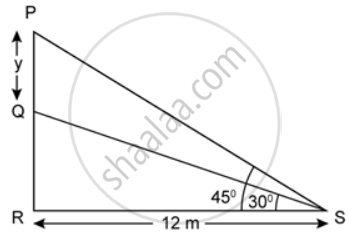
In the given figure, if tan θ = `(5)/(13), tan α = (3)/(5)` and RS = 12m, find the value of 'h'.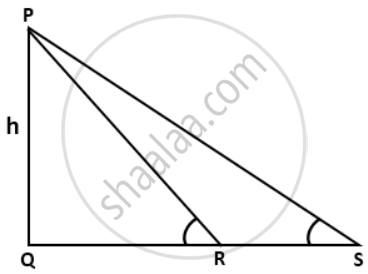
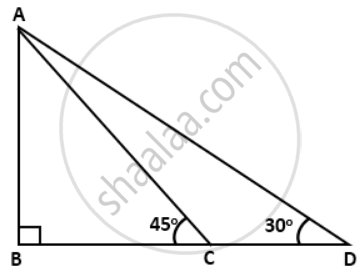
In the given figure; ∠B = 90°, ∠ADB = 30°, ∠ACB = 45° and AB = 24 m. Find the length of CD.