Advertisements
Advertisements
प्रश्न
Find the value of 'A', if 2 cos A = 1
उत्तर
2 cos A = 1
⇒ cos A = `(1)/(2)`
⇒ cos A = cos60°
⇒ A = 60°.
APPEARS IN
संबंधित प्रश्न
If 2 cos 2A = `sqrt3` and A is acute,
find:
(i) A
(ii) sin 3A
(iii) sin2 (75° - A) + cos2 (45° +A)
Use the given figure to find:
(i) tan θ°
(ii) θ°
(iii) sin2θ° - cos2θ°
(iv) Use sin θ° to find the value of x.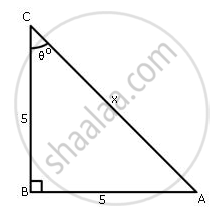
Calculate the value of A, if (cosec 2A - 2) (cot 3A - 1) = 0
If A = B = 60°, verify that: sin(A - B) = sinA cosB - cosA sinB
Find the value of: `sqrt((1 - sin^2 60°)/(1 + sin^2 60°)` If 3 tan2θ - 1 = 0, find the value
a. cosθ
b. sinθ
Find:
a. BC
b. AD
c. AC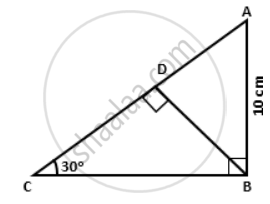
Find the value 'x', if: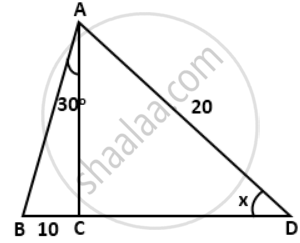
A ladder is placed against a vertical tower. If the ladder makes an angle of 30° with the ground and reaches upto a height of 18 m of the tower; find length of the ladder.
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: cos72° - cos88°
If cosθ = sin60° and θ is an acute angle find the value of 1- 2 sin2θ
