Advertisements
Advertisements
प्रश्न
Solve for 'θ': cot2(θ - 5)° = 3
उत्तर
cot2(θ - 5)° = 3
⇒ cot(θ - 5)° = `sqrt(3)`
⇒ cot(θ - 5)° = cot 30°
⇒ (θ - 5)° = 30°
⇒ θ = 30°+ 5°
⇒ θ = 35°.
APPEARS IN
संबंधित प्रश्न
If sin 3A = 1 and 0 < A < 90°, find sin A
If 2 cos 2A = `sqrt3` and A is acute,
find:
(i) A
(ii) sin 3A
(iii) sin2 (75° - A) + cos2 (45° +A)
Find the magnitude of angle A, if 2 sin A cos A - cos A - 2 sin A + 1 = 0
Find the value of 'x' in each of the following: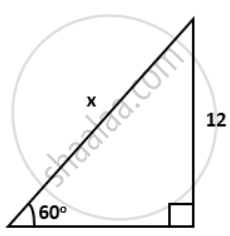
If tan x° = `(5)/(12) . tan y° = (3)/(4)` and AB = 48m; find the length CD.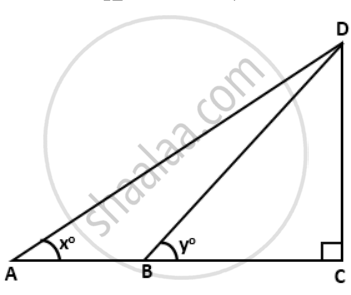
Evaluate the following: sin28° sec62° + tan49° tan41°
Evaluate the following: sec16° tan28° - cot62° cosec74°
If cos3θ = sin(θ - 34°), find the value of θ if 3θ is an acute angle.
If tan4θ = cot(θ + 20°), find the value of θ if 4θ is an acute angle.
If P, Q and R are the interior angles of ΔPQR, prove that `cot(("Q" + "R")/2) = tan "P"/(2)`
