Advertisements
Advertisements
प्रश्न
In a ΔABC median AD is produced to X such that AD = DX. Prove that ABXC is a
parallelogram.
उत्तर
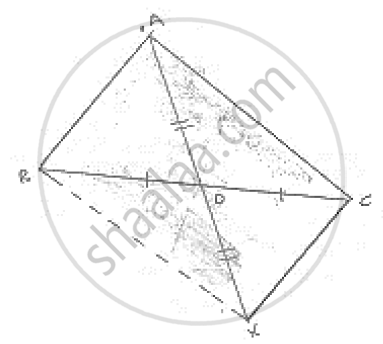
In a quadrilateral ABXC, we have
AD = DX [ Given ]
BD = DC [ Given ]
So, diagonals AX and BC bisect each other
∴ ABXC is a parallelogram
APPEARS IN
संबंधित प्रश्न
In a parallelogram ABCD, determine the sum of angles ∠C and ∠D .
In a parallelogram ABCD, if `∠`B = 135°, determine the measures of its other angles .
ABCD is a square. AC and BD intersect at O. State the measure of ∠AOB.
The sides AB and CD of a parallelogram ABCD are bisected at E and F. Prove that EBFD is a parallelogram.
In a parallelogram ABCD, write the sum of angles A and B.
In a parallelogram ABCD, the bisector of ∠A also bisects BC at X. Find AB : AD.
We get a rhombus by joining the mid-points of the sides of a
In the given Figure, if AB = 2, BC = 6, AE = 6, BF = 8, CE = 7, and CF = 7, compute the ratio of the area of quadrilateral ABDE to the area of ΔCDF. (Use congruent property of triangles)
ABCD is a square, diagonals AC and BD meet at O. The number of pairs of congruent triangles with vertex O are
Prove that the quadrilateral formed by the bisectors of the angles of a parallelogram is a rectangle.
