Advertisements
Advertisements
प्रश्न
We get a rhombus by joining the mid-points of the sides of a
विकल्प
parallelogram
rhombus
rectangle
triangle
उत्तर
We get a rhombus by joining the mid-points of the sides of a rectangle.
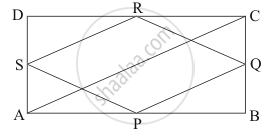
It is given a rectangle ABCD in which P,Q,R and S are the mid-points AB,BC,CD and DArespectively.
PQ,QR,RS and SP are joined.
In ΔABC, P and Q are the mid-points AB and BC respectively.
Therefore,
PQ || AC and `PQ = 1/2 AC` ……(i)
Similarly, In ΔADC,R and S are the mid-points CD and AD respectively.
Therefore,
SR || AC and `SR = 1/2 AC `……(ii)
From (i) and (ii), we get
PQ || SR and PQ || SR
Therefore,PQRS is a parallelogram. …… (iii)
Now ABCDis a rectangle.
Therefore,
AD = BC
`1/2 AD = 1/2 BC`
AS = BQ …… (iv)
In ΔAPS and ΔBPQ, we have:
AP = BP (P is the mid point of AB)
∠PAS =∠PBQ (Each is a right angle)
AS = BQ (From equation (iv))
So, by SAS congruence criteria, we get:
ΔAPS ≅ ΔBPQ
By Corresponding parts of congruent triangles property we have:
PS = PQ …… (v)
From (iii) and (v) we obtain that PQRS is a parallelogram such that RS = PQ and PQ = QR
Thus, the two adjacent sides are equal.
Thus, PQRS is a rhombus.
Hence the correct choice is (c).
APPEARS IN
संबंधित प्रश्न
In a parallelogram ABCD, determine the sum of angles ∠C and ∠D .
In a parallelogram ABCD, if `∠`B = 135°, determine the measures of its other angles .
ABCD is a square. AC and BD intersect at O. State the measure of ∠AOB.
The sides AB and CD of a parallelogram ABCD are bisected at E and F. Prove that EBFD is a parallelogram.
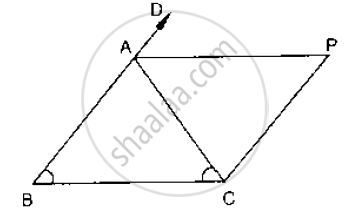
In Fig. below, AB = AC and CP || BA and AP is the bisector of exterior ∠CAD of ΔABC.
Prove that (i) ∠PAC = ∠BCA (ii) ABCP is a parallelogram
In a parallelogram ABCD, if ∠A = (3x − 20)°, ∠B = (y + 15)°, ∠C = (x + 40)°, then find the values of xand y.
In a parallelogram ABCD, the bisector of ∠A also bisects BC at X. Find AB : AD.
The figure formed by joining the mid-points of the adjacent sides of a parallelogram is a
ABCD is a parallelogram in which diagonal AC bisects ∠BAD. If ∠BAC = 35°, then ∠ABC =
ABCD is a square, diagonals AC and BD meet at O. The number of pairs of congruent triangles with vertex O are
