Advertisements
Advertisements
Question
We get a rhombus by joining the mid-points of the sides of a
Options
parallelogram
rhombus
rectangle
triangle
Solution
We get a rhombus by joining the mid-points of the sides of a rectangle.
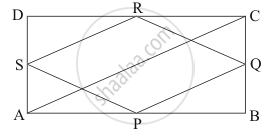
It is given a rectangle ABCD in which P,Q,R and S are the mid-points AB,BC,CD and DArespectively.
PQ,QR,RS and SP are joined.
In ΔABC, P and Q are the mid-points AB and BC respectively.
Therefore,
PQ || AC and `PQ = 1/2 AC` ……(i)
Similarly, In ΔADC,R and S are the mid-points CD and AD respectively.
Therefore,
SR || AC and `SR = 1/2 AC `……(ii)
From (i) and (ii), we get
PQ || SR and PQ || SR
Therefore,PQRS is a parallelogram. …… (iii)
Now ABCDis a rectangle.
Therefore,
AD = BC
`1/2 AD = 1/2 BC`
AS = BQ …… (iv)
In ΔAPS and ΔBPQ, we have:
AP = BP (P is the mid point of AB)
∠PAS =∠PBQ (Each is a right angle)
AS = BQ (From equation (iv))
So, by SAS congruence criteria, we get:
ΔAPS ≅ ΔBPQ
By Corresponding parts of congruent triangles property we have:
PS = PQ …… (v)
From (iii) and (v) we obtain that PQRS is a parallelogram such that RS = PQ and PQ = QR
Thus, the two adjacent sides are equal.
Thus, PQRS is a rhombus.
Hence the correct choice is (c).
APPEARS IN
RELATED QUESTIONS
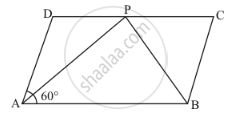
In Fig., below, ABCD is a parallelogram in which ∠A = 60°. If the bisectors of ∠A and ∠B meet at P, prove that AD = DP, PC = BC and DC = 2AD.
In a parallelogram ABCD, if `∠`B = 135°, determine the measures of its other angles .
The sides AB and CD of a parallelogram ABCD are bisected at E and F. Prove that EBFD is a parallelogram.
P and Q are the points of trisection of the diagonal BD of a parallelogram AB Prove that CQ is parallel to AP. Prove also that AC bisects PQ.
In a ΔABC median AD is produced to X such that AD = DX. Prove that ABXC is a
parallelogram.
In a parallelogram ABCD, if ∠A = (3x − 20)°, ∠B = (y + 15)°, ∠C = (x + 40)°, then find the values of xand y.
In a parallelogram ABCD, the bisector of ∠A also bisects BC at X. Find AB : AD.
ABCD is a parallelogram in which diagonal AC bisects ∠BAD. If ∠BAC = 35°, then ∠ABC =
In the given Figure, if AB = 2, BC = 6, AE = 6, BF = 8, CE = 7, and CF = 7, compute the ratio of the area of quadrilateral ABDE to the area of ΔCDF. (Use congruent property of triangles)
Prove that the quadrilateral formed by the bisectors of the angles of a parallelogram is a rectangle.
