Advertisements
Advertisements
Question
The two diagonals are equal in a
Options
parallelogram
rhombus
rectangle
trapezium
Solution
Two diagonals are equal only in a rectangle.
This can be proved as follows:
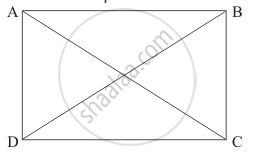
The rectangle is given as ABCD, with the two diagonals as AD and BC.
In ΔADB and ΔBCD:
AD = BC (Opposite sides of a rectangle are equal.)
CD = CD (Common)
∠ADC = ∠BCD (Each angle in a rectangle is a right angle)
Thus,
ΔADB ≅ ΔBCD (By SAS Congruence Rule)
By Corresponding parts of congruent triangles property we have:
AC = BD
Therefore, in a rectangle the two diagonals are equal.
Hence the correct choice is (c).
APPEARS IN
RELATED QUESTIONS
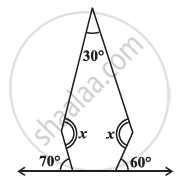
Find the angle measure x in the given Figure
Two opposite angles of a parallelogram are (3x – 2)° and (50 – x)°. Find the measure of each angle of the parallelogram .
If an angle of a parallelogram is two-third of its adjacent angle, find the angles of the parallelogram .
If the angles of a quadrilateral are in the ratio 3 : 5 : 9 : 13, then find the measure of the smallest angle.
The diagonals of a rectangle ABCD meet at O, If ∠BOC = 44°, find ∠OAD.
In the given figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD = 146°, find ∠AOB.
If an angle of a parallelogram is two-third of its adjacent angle, the smallest angle of the parallelogram is
If the diagonals of a rhombus are 18 cm and 24 cm respectively, then its side is equal to
The angles P, Q, R and S of a quadrilateral are in the ratio 1:3:7:9. Then PQRS is a ______.
Three angles of a quadrilateral are equal. Fourth angle is of measure 120°. What is the measure of equal angles?
