Topics
Number Systems
Number Systems
Polynomials
Algebra
Coordinate Geometry
Linear Equations in Two Variables
Geometry
Coordinate Geometry
Introduction to Euclid’S Geometry
Mensuration
Statistics and Probability
Lines and Angles
- Introduction to Lines and Angles
- Basic Terms and Definitions
- Intersecting Lines and Non-intersecting Lines
- Introduction to Parallel Lines
- Pairs of Angles
- Parallel Lines and a Transversal
- Angle Sum Property of a Triangle
Triangles
- Concept of Triangles
- Congruence of Triangles
- Criteria for Congruence of Triangles
- Properties of a Triangle
- Some More Criteria for Congruence of Triangles
- Inequalities in a Triangle
Quadrilaterals
- Concept of Quadrilaterals
- Properties of a Quadrilateral
- Types of Quadrilaterals
- Another Condition for a Quadrilateral to Be a Parallelogram
- Theorem of Midpoints of Two Sides of a Triangle
- Property: The Opposite Sides of a Parallelogram Are of Equal Length.
- Theorem: A Diagonal of a Parallelogram Divides It into Two Congruent Triangles.
- Theorem : If Each Pair of Opposite Sides of a Quadrilateral is Equal, Then It is a Parallelogram.
- Property: The Opposite Angles of a Parallelogram Are of Equal Measure.
- Theorem: If in a Quadrilateral, Each Pair of Opposite Angles is Equal, Then It is a Parallelogram.
- Property: The diagonals of a parallelogram bisect each other. (at the point of their intersection)
- Theorem : If the Diagonals of a Quadrilateral Bisect Each Other, Then It is a Parallelogram
Circles
Areas - Heron’S Formula
Surface Areas and Volumes
Statistics
Algebraic Expressions
Algebraic Identities
Area
Constructions
- Introduction of Constructions
- Basic Constructions
- Some Constructions of Triangles
Probability
Theorem
Theorem: A diagonal of a parallelogram divides it into two congruent triangles.
Proof : Let ABCD be a parallelogram and AC be a diagonal in following fig. 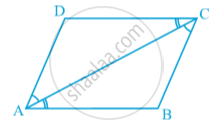
Observe that the diagonal AC divides parallelogram ABCD into two triangles, namely, ∆ ABC and ∆ CDA. We need to prove that these triangles are congruent.
In ∆ ABC and ∆ CDA, note that BC || AD and AC is a transversal.
So, ∠ BCA = ∠ DAC (Pair of alternate angles)
Also, AB || DC and AC is a transversal.
So, ∠ BAC = ∠ DCA (Pair of alternate angles)
and AC = CA (Common)
So, ∆ ABC ≅ ∆ CDA (ASA rule)
or, diagonal AC divides parallelogram ABCD into two congruent triangles ABC and CDA.
Now, measure the opposite sides of parallelogram ABCD.
You will find that AB = DC and AD = BC.
If you would like to contribute notes or other learning material, please submit them using the button below.
Shaalaa.com | Theorem - Diagonal divides parallelogram into two congruent triangles
to track your progress
