Advertisements
Advertisements
Question
P and Q are the points of trisection of the diagonal BD of a parallelogram AB Prove that CQ is parallel to AP. Prove also that AC bisects PQ.
Solution
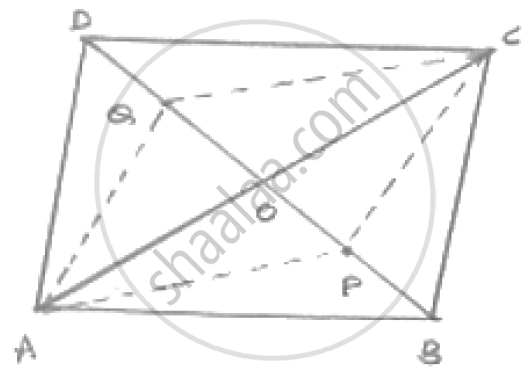
We know that, diagonals of a parallelogram bisect each other
∴OA = OC and OB = OD
Since P and Q are point of intersection of BD
∴BP = PQ = QD
Now, OB = OD and BP = QD
⇒ OB - BP = OD - QD
⇒ OP = OQ
Thus in quadrilateral APCQ, we have
OA = OC and OP = OQ
⇒ diagonals of quadrilateral APCQ bisect each other
∴APCQ is a parallelogram
Hence AP || CQ
APPEARS IN
RELATED QUESTIONS
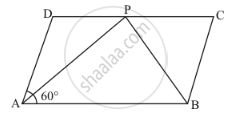
In Fig., below, ABCD is a parallelogram in which ∠A = 60°. If the bisectors of ∠A and ∠B meet at P, prove that AD = DP, PC = BC and DC = 2AD.
In a parallelogram ABCD, if `∠`B = 135°, determine the measures of its other angles .
ABCD is a square. AC and BD intersect at O. State the measure of ∠AOB.
In a ΔABC median AD is produced to X such that AD = DX. Prove that ABXC is a
parallelogram.
In a parallelogram ABCD, the bisector of ∠A also bisects BC at X. Find AB : AD.
The figure formed by joining the mid-points of the adjacent sides of a rhombus is a
The figure formed by joining the mid-points of the adjacent sides of a parallelogram is a
In a quadrilateral ABCD, ∠A + ∠C is 2 times ∠B + ∠D. If ∠A = 140° and ∠D = 60°, then ∠B=
ABCD is a square, diagonals AC and BD meet at O. The number of pairs of congruent triangles with vertex O are
Prove that the quadrilateral formed by the bisectors of the angles of a parallelogram is a rectangle.
