Advertisements
Advertisements
Question
ABCD is a square, diagonals AC and BD meet at O. The number of pairs of congruent triangles with vertex O are
Options
6
8
4
12
Solution
ABCD is a square, diagonals AC and BD meet at O. The number of pairs of congruent triangles with vertex O are 6
APPEARS IN
RELATED QUESTIONS
In a parallelogram ABCD, determine the sum of angles ∠C and ∠D .
In a ΔABC median AD is produced to X such that AD = DX. Prove that ABXC is a
parallelogram.
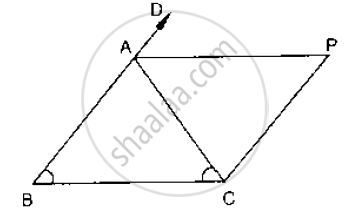
In Fig. below, AB = AC and CP || BA and AP is the bisector of exterior ∠CAD of ΔABC.
Prove that (i) ∠PAC = ∠BCA (ii) ABCP is a parallelogram
In a parallelogram ABCD, write the sum of angles A and B.
In a parallelogram ABCD, if ∠D = 115°, then write the measure of ∠A.
In a parallelogram ABCD, if ∠A = (3x − 20)°, ∠B = (y + 15)°, ∠C = (x + 40)°, then find the values of xand y.
PQRS is a quadrilateral, PR and QS intersect each other at O. In which of the following case, PQRS is a parallelogram?
∠P = 100°, ∠Q = 80°, ∠R = 95°
ABCD is a parallelogram in which diagonal AC bisects ∠BAD. If ∠BAC = 35°, then ∠ABC =
In the given Figure, if AB = 2, BC = 6, AE = 6, BF = 8, CE = 7, and CF = 7, compute the ratio of the area of quadrilateral ABDE to the area of ΔCDF. (Use congruent property of triangles)
Prove that the quadrilateral formed by the bisectors of the angles of a parallelogram is a rectangle.
