Topics
Number Systems
Number Systems
Polynomials
Algebra
Coordinate Geometry
Linear Equations in Two Variables
Geometry
Coordinate Geometry
Introduction to Euclid’S Geometry
Mensuration
Statistics and Probability
Lines and Angles
- Introduction to Lines and Angles
- Basic Terms and Definitions
- Intersecting Lines and Non-intersecting Lines
- Introduction to Parallel Lines
- Pairs of Angles
- Parallel Lines and a Transversal
- Angle Sum Property of a Triangle
Triangles
- Concept of Triangles
- Congruence of Triangles
- Criteria for Congruence of Triangles
- Properties of a Triangle
- Some More Criteria for Congruence of Triangles
- Inequalities in a Triangle
Quadrilaterals
- Concept of Quadrilaterals
- Properties of a Quadrilateral
- Types of Quadrilaterals
- Another Condition for a Quadrilateral to Be a Parallelogram
- Theorem of Midpoints of Two Sides of a Triangle
- Property: The Opposite Sides of a Parallelogram Are of Equal Length.
- Theorem: A Diagonal of a Parallelogram Divides It into Two Congruent Triangles.
- Theorem : If Each Pair of Opposite Sides of a Quadrilateral is Equal, Then It is a Parallelogram.
- Property: The Opposite Angles of a Parallelogram Are of Equal Measure.
- Theorem: If in a Quadrilateral, Each Pair of Opposite Angles is Equal, Then It is a Parallelogram.
- Property: The diagonals of a parallelogram bisect each other. (at the point of their intersection)
- Theorem : If the Diagonals of a Quadrilateral Bisect Each Other, Then It is a Parallelogram
Circles
Areas - Heron’S Formula
Surface Areas and Volumes
Statistics
Algebraic Expressions
Algebraic Identities
Area
Constructions
- Introduction of Constructions
- Basic Constructions
- Some Constructions of Triangles
Probability
Notes
The diagonals of a parallelogram bisect each other.
Given: ABCD is a parallelogram.
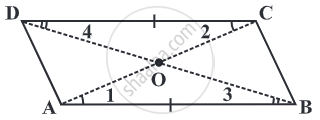
To Prove: AO = OC and BO = OD.
Construction: Draw diagonal AC and diagonal BD.
Proof:
ABCD is a parallelogram.
∴ AB || DC and AD || BC
Now,
AB || DC, AC is the transversal intersecting them at A and C respectively.
∠BAC = ∠DCA ........(Alternate interior angles)
Thus, ∠BAO = ∠ DCO .........(1)
As, AB || DC and BD is the transversal intersecting them at B and D respectively.
∠ABD = ∠CDB ........(Alternate interior angles)
So, ∠ABO = ∠CDO ...(2)
In △ AOB and △ COD,
∠ DCO ≅ ∠BAO .....(Pair of alternate interior angles)(1)
∠ CDO ≅ ∠ABO .....(Pair of alternate interior angles)(2)
side DC = side AB ....(parallel side)
by ASA congruency condition,
△ AOB ≅ △ COD.
This gives, AO = CO and BO = DO.....(C.P.C.T)
Hence proved.
Example
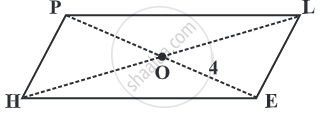
If OE = 4 then OP also is 4. .......(The diagonals of a parallelogram bisect each other.)
So, PE = 8,
Therefore, HL = 8 + 5 = 13
Hence, OH = `1/2 xx 13` = 6.5 cms.