Advertisements
Advertisements
Question
The diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If ∠DAC = 32º and ∠AOB = 70º, then ∠DBC is equal to ______.
Options
24º
86º
38º
32º
Solution
The diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If ∠DAC = 32º and ∠AOB = 70º, then ∠DBC is equal to 38º.
Explanation:
Given: ∠AOB = 70°
∠DAC = 32°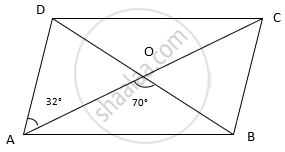
∵ AD || BC and AC is transversal
∴ ∠ACB = 32°
Now, ∠AOB + ∠BOC = 180°
⇒ 70° + ∠BOC = 180°
⇒ ∠BOC = 180° – 70°
⇒ ∠BOC = 110°
Sum of all angles of a triangle = 180°
⇒ ∠BOC + ∠BCO + ∠OBC = 180°
⇒ 110° + 32° + ∠OBC = 180°
⇒ 142° + ∠OBC = 180°
⇒ ∠OBC = 180° – 142°
⇒ ∠OBC = 38°
Hence, ∠DBC = 38°
APPEARS IN
RELATED QUESTIONS
Diagonals of a parallelogram `square`WXYZ intersect each other at point O. If ∠XYZ = 135° then what is the measure of ∠XWZ and ∠YZW?
If l(OY)= 5 cm then l(WY)= ?
Diagonals AC and BD of a parallelogram ABCD intersect each other at O. If OA = 3 cm and OD = 2 cm, determine the lengths of AC and BD.
Diagonals of a parallelogram are perpendicular to each other. Is this statement true? Give reason for your answer.
E and F are points on diagonal AC of a parallelogram ABCD such that AE = CF. Show that BFDE is a parallelogram.
In a parallelogram ABCD, AB = 10 cm and AD = 6 cm. The bisector of ∠A meets DC in E. AE and BC produced meet at F. Find the length of CF.
In the following figure, AB || DE, AB = DE, AC || DF and AC = DF. Prove that BC || EF and BC = EF.

ABCD is a rectangle in which diagonal BD bisects ∠B. Show that ABCD is a square.
If diagonals of a quadrilateral bisect each other, it must be a parallelogram.
The point of intersection of diagonals of a quadrilateral divides one diagonal in the ratio 1:2. Can it be a parallelogram? Why or why not?
Two sticks each of length 5 cm are crossing each other such that they bisect each other. What shape is formed by joining their endpoints? Give reason.
