NCERT Exemplar solutions for Mathematics [English] Class 9 chapter 8 - Quadrilaterals [Latest edition]

Three angles of a quadrilateral are 75º, 90º and 75º. The fourth angle is ______.

A diagonal of a rectangle is inclined to one side of the rectangle at 25º. The acute angle between the diagonals is ______.

ABCD is a rhombus such that ∠ACB = 40º. Then ∠ADB is ______.

The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rectangle, if ______.

The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if ______.

If angles A, B, C and D of the quadrilateral ABCD, taken in order, are in the ratio 3 : 7 : 6 : 4, then ABCD is a ______.

If bisectors of ∠A and ∠B of a quadrilateral ABCD intersect each other at P, of ∠B and ∠C at Q, of ∠C and ∠D at R and of ∠D and ∠A at S, then PQRS is a ______.

If APB and CQD are two parallel lines, then the bisectors of the angles APQ, BPQ, CQP and PQD form ______.

The figure obtained by joining the mid-points of the sides of a rhombus, taken in order, is ______.

D and E are the mid-points of the sides AB and AC of ∆ABC and O is any point on side BC. O is joined to A. If P and Q are the mid-points of OB and OC respectively, then DEQP is ______.

The figure formed by joining the mid-points of the sides of a quadrilateral ABCD, taken in order, is a square only if, ______.

The diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If ∠DAC = 32º and ∠AOB = 70º, then ∠DBC is equal to ______.

Which of the following is not true for a parallelogram?

D and E are the mid-points of the sides AB and AC respectively of ∆ABC. DE is produced to F. To prove that CF is equal and parallel to DA, we need an additional information which is ______.

Diagonals AC and BD of a parallelogram ABCD intersect each other at O. If OA = 3 cm and OD = 2 cm, determine the lengths of AC and BD.

Diagonals of a parallelogram are perpendicular to each other. Is this statement true? Give reason for your answer.

Can the angles 110º, 80º, 70º and 95º be the angles of a quadrilateral? Why or why not?

In quadrilateral ABCD, ∠A + ∠D = 180º. What special name can be given to this quadrilateral?

All the angles of a quadrilateral are equal. What special name is given to this quadrilateral?

Diagonals of a rectangle are equal and perpendicular. Is this statement true? Give reason for your answer.

Can all the four angles of a quadrilateral be obtuse angles? Give reason for your answer.

In ∆ABC, AB = 5 cm, BC = 8 cm and CA = 7 cm. If D and E are respectively the mid-points of AB and BC, determine the length of DE.

In the following figure, it is given that BDEF and FDCE are parallelograms. Can you say that BD = CD? Why or why not?

In the following figure, ABCD and AEFG are two parallelograms. If ∠C = 55º, determine ∠F.

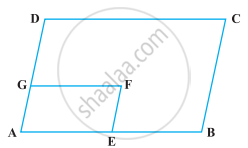
Can all the angles of a quadrilateral be acute angles? Give reason for your answer.

Can all the angles of a quadrilateral be right angles? Give reason for your answer.

Diagonals of a quadrilateral ABCD bisect each other. If ∠A = 35º, determine ∠B.

Opposite angles of a quadrilateral ABCD are equal. If AB = 4 cm, determine CD.

One angle of a quadrilateral is of 108º and the remaining three angles are equal. Find each of the three equal angles.

ABCD is a trapezium in which AB || DC and ∠A = ∠B = 45º. Find angles C and D of the trapezium.

The angle between two altitudes of a parallelogram through the vertex of an obtuse angle of the parallelogram is 60º. Find the angles of the parallelogram.

ABCD is a rhombus in which altitude from D to side AB bisects AB. Find the angles of the rhombus.

E and F are points on diagonal AC of a parallelogram ABCD such that AE = CF. Show that BFDE is a parallelogram.

E is the mid-point of the side AD of the trapezium ABCD with AB || DC. A line through E drawn parallel to AB intersect BC at F. Show that F is the mid-point of BC. [Hint: Join AC]

Through A, B and C, lines RQ, PR and QP have been drawn, respectively parallel to sides BC, CA and AB of a ∆ABC as shown in the following figure. Show that BC = `1/2` QR.

D, E and F are the mid-points of the sides BC, CA and AB, respectively of an equilateral triangle ABC. Show that ∆DEF is also an equilateral triangle.

Points P and Q have been taken on opposite sides AB and CD, respectively of a parallelogram ABCD such that AP = CQ (Figure). Show that AC and PQ bisect each other.

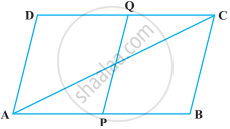
In the following figure, P is the mid-point of side BC of a parallelogram ABCD such that ∠BAP = ∠DAP. Prove that AD = 2CD.

A square is inscribed in an isosceles right triangle so that the square and the triangle have one angle common. Show that the vertex of the square opposite the vertex of the common angle bisects the hypotenuse.

In a parallelogram ABCD, AB = 10 cm and AD = 6 cm. The bisector of ∠A meets DC in E. AE and BC produced meet at F. Find the length of CF.

P, Q, R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.

P, Q, R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD such that AC ⊥ BD. Prove that PQRS is a rectangle.

P, Q, R and S are respectively the mid-points of sides AB, BC, CD and DA of quadrilateral ABCD in which AC = BD and AC ⊥ BD. Prove that PQRS is a square.

A diagonal of a parallelogram bisects one of its angles. Show that it is a rhombus.

P and Q are the mid-points of the opposite sides AB and CD of a parallelogram ABCD. AQ intersects DP at S and BQ intersects CP at R. Show that PRQS is a parallelogram.

ABCD is a quadrilateral in which AB || DC and AD = BC. Prove that ∠A = ∠B and ∠C = ∠D.

In the following figure, AB || DE, AB = DE, AC || DF and AC = DF. Prove that BC || EF and BC = EF.

E is the mid-point of a median AD of ∆ABC and BE is produced to meet AC at F. Show that AF = `1/3` AC.

Show that the quadrilateral formed by joining the mid-points of the consecutive sides of a square is also a square.

E and F are respectively the mid-points of the non-parallel sides AD and BC of a trapezium ABCD. Prove that EF || AB and EF = `1/2` (AB + CD).

[Hint: Join BE and produce it to meet CD produced at G.]

Prove that the quadrilateral formed by the bisectors of the angles of a parallelogram is a rectangle.

P and Q are points on opposite sides AD and BC of a parallelogram ABCD such that PQ passes through the point of intersection O of its diagonals AC and BD. Show that PQ is bisected at O.

ABCD is a rectangle in which diagonal BD bisects ∠B. Show that ABCD is a square.

D, E and F are respectively the mid-points of the sides AB, BC and CA of a triangle ABC. Prove that by joining these mid-points D, E and F, the triangles ABC is divided into four congruent triangles.

Prove that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of the trapezium.

P is the mid-point of the side CD of a parallelogram ABCD. A line through C parallel to PA intersects AB at Q and DA produced at R. Prove that DA = AR and CQ = QR.