Advertisements
Advertisements
Question
Prove that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of the trapezium.
Solution
Given: Let ABCD be a trapezium in which AB || DC and let M and N be the mid-points of the diagonals AC and BD, respectively.
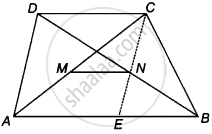
To prove: MN || AB || CD
Construction: Join CN and produce it to meet AB at E.
In ΔCDN and ΔEBN, we have
DN = BN ...[Since, N is the mid-point of BD]
∠DCN = ∠BEN ...[Alternate interior angles]
And ∠CDN = ∠EBN ...[Alternate interior angles]
∴ ΔCDN ≅ ΔEBN ...[By AAS congruence rule]
∴ DC = EB and CN = NE ...[By CPCT rule]
Thus, in ΔCAE, the points M and N are the mid-points of AC and CE, respectively.
∴ MN || AE ...[By mid-point theorem]
⇒ MN || AB || CD
Hence proved.
APPEARS IN
RELATED QUESTIONS
ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
Fill in the blank to make the following statement correct
The triangle formed by joining the mid-points of the sides of an isosceles triangle is
In the adjacent figure, `square`ABCD is a trapezium AB || DC. Points M and N are midpoints of diagonal AC and DB respectively then prove that MN || AB.

Prove that the figure obtained by joining the mid-points of the adjacent sides of a rectangle is a rhombus.
In the figure, give below, 2AD = AB, P is mid-point of AB, Q is mid-point of DR and PR // BS. Prove that:
(i) AQ // BS
(ii) DS = 3 Rs.

In triangle ABC, angle B is obtuse. D and E are mid-points of sides AB and BC respectively and F is a point on side AC such that EF is parallel to AB. Show that BEFD is a parallelogram.
In ΔABC, D, E, F are the midpoints of BC, CA and AB respectively. Find ∠FDB if ∠ACB = 115°.
If L and M are the mid-points of AB, and DC respectively of parallelogram ABCD. Prove that segment DL and BM trisect diagonal AC.
In AABC, D and E are two points on the side AB such that AD = DE = EB. Through D and E, lines are drawn parallel to BC which meet the side AC at points F and G respectively. Through F and G, lines are drawn parallel to AB which meet the side BC at points M and N respectively. Prove that BM = MN = NC.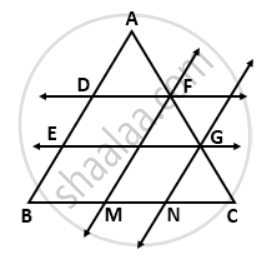
D, E and F are the mid-points of the sides BC, CA and AB, respectively of an equilateral triangle ABC. Show that ∆DEF is also an equilateral triangle.
