Advertisements
Advertisements
Question
ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
Solution
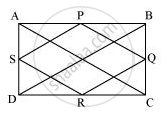
Let us join AC and BD.
In ΔABC,
P and Q are the mid-points of AB and BC respectively.
∴ PQ || AC and PQ = `1/2 AC` ...(Mid-point theorem) ...(1)
Similarly, in ΔADC,
SR || AC and SR = `1/2 AC` ...(Mid-point theorem) ...(2)
Clearly, PQ || SR and PQ = SR
Since in quadrilateral PQRS, one pair of opposite sides is equal and parallel to each other, it is a parallelogram.
∴ PS || QR and PS = QR ...(Opposite sides of the parallelogram) ...(3)
In ΔBCD, Q and R are the mid-points of side BC and CD respectively.
∴ QR || BD and QR = `1/2 BD` ...(Mid-point theorem) ...(4)
However, the diagonals of a rectangle are equal.
∴ AC = BD …(5)
By using equation (1), (2), (3), (4), and (5), we obtain
PQ = QR = SR = PS
Therefore, PQRS is a rhombus.
APPEARS IN
RELATED QUESTIONS
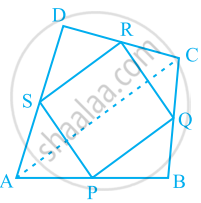
ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see the given figure). AC is a diagonal. Show that:
- SR || AC and SR = `1/2AC`
- PQ = SR
- PQRS is a parallelogram.
In the given figure, seg PD is a median of ΔPQR. Point T is the mid point of seg PD. Produced QT intersects PR at M. Show that `"PM"/"PR" = 1/3`.
[Hint: DN || QM]

In the adjacent figure, `square`ABCD is a trapezium AB || DC. Points M and N are midpoints of diagonal AC and DB respectively then prove that MN || AB.

ABCD is a quadrilateral in which AD = BC. E, F, G and H are the mid-points of AB, BD, CD and Ac respectively. Prove that EFGH is a rhombus.

In a triangle ABC, AD is a median and E is mid-point of median AD. A line through B and E meets AC at point F.
Prove that: AC = 3AF.
In parallelogram ABCD, E and F are mid-points of the sides AB and CD respectively. The line segments AF and BF meet the line segments ED and EC at points G and H respectively.
Prove that:
(i) Triangles HEB and FHC are congruent;
(ii) GEHF is a parallelogram.
In ΔABC, AB = 12 cm and AC = 9 cm. If M is the mid-point of AB and a straight line through M parallel to AC cuts BC in N, what is the length of MN?
Side AC of a ABC is produced to point E so that CE = `(1)/(2)"AC"`. D is the mid-point of BC and ED produced meets AB at F. Lines through D and C are drawn parallel to AB which meets AC at point P and EF at point R respectively. Prove that: 4CR = AB.
In ΔABC, D, E and F are the midpoints of AB, BC and AC.
Show that AE and DF bisect each other.
The figure obtained by joining the mid-points of the sides of a rhombus, taken in order, is ______.
