Advertisements
Advertisements
Question
In the given figure, seg PD is a median of ΔPQR. Point T is the mid point of seg PD. Produced QT intersects PR at M. Show that `"PM"/"PR" = 1/3`.
[Hint: DN || QM]

Solution
Given: seg PD is a median of ΔPQR. Point T is the mid point of seg PD.
To prove: `"PM"/"PR" = 1/3`
Construction: Draw seg DN || seg QM such that P-M-N and M-N-R.
Proof:
In ΔPDN,
Point T is the mid-point of seg PD. ...(Given)
seg TM || seg DN ...(Construction)
∴ Point M is the mid-point of seg PN. ...(Converse of mid-point theorem)[P-M-N]
∴ PM = MN ...(i)
In ΔQMR,
Point D is the mid-point of seg QR. ...(Given)
seg DN || seg QM. ...(Construction)
∴ Point N is the mid-point of seg MR. ...(Converse of mid-point theorem)[M-N-R]
∴ RN = MN ...(ii)
PM = MN = RN ...[From (i) and (ii)] ...(iii)
Now,
PR = PM + MN + RN
∴ PR = PM + PM + PM ...[From (iii)]
∴ PR = 3PM
∴ `"PM"/"PR" = 1/3`
Hence proved.
APPEARS IN
RELATED QUESTIONS
ABC is a triangle and through A, B, C lines are drawn parallel to BC, CA and AB respectively
intersecting at P, Q and R. Prove that the perimeter of ΔPQR is double the perimeter of
ΔABC
BM and CN are perpendiculars to a line passing through the vertex A of a triangle ABC. If
L is the mid-point of BC, prove that LM = LN.
D and F are midpoints of sides AB and AC of a triangle ABC. A line through F and parallel to AB meets BC at point E.
- Prove that BDFE is a parallelogram
- Find AB, if EF = 4.8 cm.
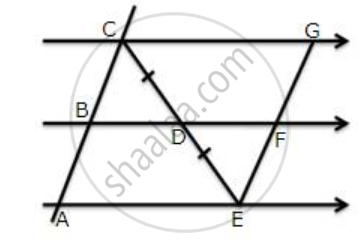
Use the following figure to find:
(i) BC, if AB = 7.2 cm.
(ii) GE, if FE = 4 cm.
(iii) AE, if BD = 4.1 cm
(iv) DF, if CG = 11 cm.
In trapezium ABCD, sides AB and DC are parallel to each other. E is mid-point of AD and F is mid-point of BC.
Prove that: AB + DC = 2EF.
In triangle ABC ; D and E are mid-points of the sides AB and AC respectively. Through E, a straight line is drawn parallel to AB to meet BC at F.
Prove that BDEF is a parallelogram. If AB = 16 cm, AC = 12 cm and BC = 18 cm,
find the perimeter of the parallelogram BDEF.
In parallelogram ABCD, P is the mid-point of DC. Q is a point on AC such that CQ = `(1)/(4)"AC"`. PQ produced meets BC at R. Prove that
(i) R is the mid-point of BC, and
(ii) PR = `(1)/(2)"DB"`.
In ΔABC, P is the mid-point of BC. A line through P and parallel to CA meets AB at point Q, and a line through Q and parallel to BC meets median AP at point R. Prove that: BC = 4QR
ABCD is a parallelogram.E is the mid-point of CD and P is a point on AC such that PC = `(1)/(4)"AC"`. EP produced meets BC at F. Prove that: 2EF = BD.
In ΔABC, D and E are the midpoints of the sides AB and AC respectively. F is any point on the side BC. If DE intersects AF at P show that DP = PE.
