Advertisements
Advertisements
Question
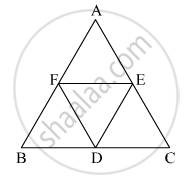
In the given figure, ΔABC is an equilateral traingle. Points F, D and E are midpoints of side AB, side BC, side AC respectively. Show that ΔFED is an equilateral traingle.
Solution
Given: ∆ABC is an equilateral triangle and D, E and F are mid-points of BC, AC and AB respectively.
To prove: ∆FED is an equilateral triangle.
Proof:
In ΔABC,
Points F and E are the midpoints of sides AB and AC respectively. ...(Given)
∴ FE = `1/2` BC ...(From midpoint theorem) ...(i)
In ΔABC,
Points D and E are the midpoints of sides BC and AC respectively. ...(Given)
∴ DE = `1/2` AB ...(From midpoint theorem) ...(ii)
In ΔABC,
Points D and F are the midpoints of sides BC and AB respectively. ...(Given)
∴ DF = `1/2` AC ...(From midpoint theorem) ...(iii)
Now, ΔABC is an equilateral triangle.
∴ BC = AB = AC ...(Sides of equilateral triangle)
∴ `1/2` BC = `1/2` AB = `1/2` AC ...(Multiplying both sides by `1 /2`)
∴ FE = DE = DF ...[From (i), (ii) and (iii)]
∴ ΔFED is an equilateral triangle.
APPEARS IN
RELATED QUESTIONS
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that
- D is the mid-point of AC
- MD ⊥ AC
- CM = MA = `1/2AB`
ABCD is a rhombus, EABF is a straight line such that EA = AB = BF. Prove that ED and FC when produced meet at right angles
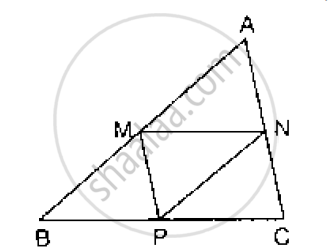
In Fig. below, M, N and P are the mid-points of AB, AC and BC respectively. If MN = 3 cm, NP = 3.5 cm and MP = 2.5 cm, calculate BC, AB and AC.
Fill in the blank to make the following statement correct
The triangle formed by joining the mid-points of the sides of an isosceles triangle is
D, E, and F are the mid-points of the sides AB, BC and CA of an isosceles ΔABC in which AB = BC.
Prove that ΔDEF is also isosceles.
In parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P.
Prove that:
(i) BP = 2AD
(ii) O is the mid-point of AP.
In parallelogram ABCD, E and F are mid-points of the sides AB and CD respectively. The line segments AF and BF meet the line segments ED and EC at points G and H respectively.
Prove that:
(i) Triangles HEB and FHC are congruent;
(ii) GEHF is a parallelogram.
Side AC of a ABC is produced to point E so that CE = `(1)/(2)"AC"`. D is the mid-point of BC and ED produced meets AB at F. Lines through D and C are drawn parallel to AB which meets AC at point P and EF at point R respectively. Prove that: 4CR = AB.
D and E are the mid-points of the sides AB and AC of ∆ABC and O is any point on side BC. O is joined to A. If P and Q are the mid-points of OB and OC respectively, then DEQP is ______.
P and Q are the mid-points of the opposite sides AB and CD of a parallelogram ABCD. AQ intersects DP at S and BQ intersects CP at R. Show that PRQS is a parallelogram.
