Advertisements
Advertisements
Question
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that
- D is the mid-point of AC
- MD ⊥ AC
- CM = MA = `1/2AB`
Solution
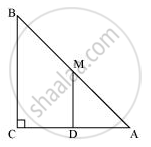
(i) In ΔABC,
It is given that M is the mid-point of AB and MD || BC.
Therefore, D is the mid-point of AC. ...(Converse of mid-point theorem)
(ii) As DM || CB and AC is a transversal line for them, therefore,
∠MDC + ∠DCB = 180° ...(Co-interior angles)
∠MDC + 90° = 180°
∠MDC = 90°
∴ MD ⊥ AC
(iii) Join MC.
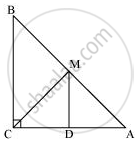
In ΔAMD and ΔCMD,
AD = CD ...(D is the mid-point of side AC)
∠ADM = ∠CDM ...(Each 90º)
DM = DM (Common)
∴ ΔAMD ≅ ΔCMD ...(By SAS congruence rule)
Therefore, AM = CM ...(By CPCT)
However, AM = `1/2AB` ...(M is the mid-point of AB)
Therefore, it can be said that
CM = AM = `1/2AB`
APPEARS IN
RELATED QUESTIONS
In a triangle, P, Q and R are the mid-points of sides BC, CA and AB respectively. If AC =
21 cm, BC = 29 cm and AB = 30 cm, find the perimeter of the quadrilateral ARPQ.
ABCD is a parallelogram, E and F are the mid-points of AB and CD respectively. GH is any line intersecting AD, EF and BC at G, P and H respectively. Prove that GP = PH
In the given figure, seg PD is a median of ΔPQR. Point T is the mid point of seg PD. Produced QT intersects PR at M. Show that `"PM"/"PR" = 1/3`.
[Hint: DN || QM]

The following figure shows a trapezium ABCD in which AB // DC. P is the mid-point of AD and PR // AB. Prove that:
PR = `[1]/[2]` ( AB + CD)

A parallelogram ABCD has P the mid-point of Dc and Q a point of Ac such that
CQ = `[1]/[4]`AC. PQ produced meets BC at R.

Prove that
(i)R is the midpoint of BC
(ii) PR = `[1]/[2]` DB
Use the following figure to find:
(i) BC, if AB = 7.2 cm.
(ii) GE, if FE = 4 cm.
(iii) AE, if BD = 4.1 cm
(iv) DF, if CG = 11 cm.
In ΔABC, BE and CF are medians. P is a point on BE produced such that BE = EP and Q is a point on CF produced such that CF = FQ. Prove that: A is the mid-point of PQ.
The diagonals of a quadrilateral intersect each other at right angle. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is a rectangle.
In ΔABC, X is the mid-point of AB, and Y is the mid-point of AC. BY and CX are produced and meet the straight line through A parallel to BC at P and Q respectively. Prove AP = AQ.
Prove that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of the trapezium.
