Advertisements
Advertisements
Question
In ΔABC, BE and CF are medians. P is a point on BE produced such that BE = EP and Q is a point on CF produced such that CF = FQ. Prove that: A is the mid-point of PQ.
Solution
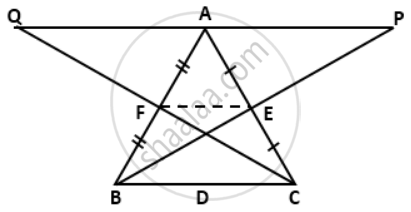
Since BE and CF are medians, F is the mid-point of AB and E is the mid-point of AC. Now, the line joining the mid-point of any two sides is parallel and half of the third side, we have In ΔACQ,
EF || AQ and EF = `(1)/(2)"AQ"` ....(i)
In ΔABP,
EF || AP and EF = `(1)/(2)"AP"` ....(ii)
From (i) and (ii)
`"EF" = (1)/(2)"AQ" and "EF" = (1)/(2)"AP"`
⇒ `(1)/(2)"AQ" = (1)/(2)"AP"`
⇒ AQ = AP
⇒ A is the mid-point of QP.
APPEARS IN
RELATED QUESTIONS
Show that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other.
In a triangle, P, Q and R are the mid-points of sides BC, CA and AB respectively. If AC =
21 cm, BC = 29 cm and AB = 30 cm, find the perimeter of the quadrilateral ARPQ.
In a ΔABC, BM and CN are perpendiculars from B and C respectively on any line passing
through A. If L is the mid-point of BC, prove that ML = NL.
In below Fig, ABCD is a parallelogram in which P is the mid-point of DC and Q is a point on AC such that CQ = `1/4` AC. If PQ produced meets BC at R, prove that R is a mid-point of BC.

Fill in the blank to make the following statement correct:
The figure formed by joining the mid-points of consecutive sides of a quadrilateral is
In triangle ABC, AD is the median and DE, drawn parallel to side BA, meets AC at point E.
Show that BE is also a median.
L and M are the mid-point of sides AB and DC respectively of parallelogram ABCD. Prove that segments DL and BM trisect diagonal AC.
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if ______.
P and Q are the mid-points of the opposite sides AB and CD of a parallelogram ABCD. AQ intersects DP at S and BQ intersects CP at R. Show that PRQS is a parallelogram.
Show that the quadrilateral formed by joining the mid-points of the consecutive sides of a square is also a square.
