Advertisements
Advertisements
Question
In the given figure, `square`PQRS and `square`MNRL are rectangles. If point M is the midpoint of side PR then prove that,
- SL = LR
- LN = `1/2`SQ

Solution
(i) `square`LMNR and `square`MNRL are rectangles.
∴ Side LM || Side RN ...(Opposite sides of rectangle)
That is, Side LM || Side RQ ...(R-N-Q) ...(i)
Side RQ || Side SP ...(Opposite sides of the rectangle) ...(ii)
From (i) and (ii),
Side LM || Side SP ...(iii)
In ΔRSP,
Point M is the midpoint of Seg PR.
Line LM || Line SP ...[From (iii)]
∴ Point L is the midpoint of Seg SR. ...(Converse of Midpoint Theorem) ...(iv)
∴ SL = LR
(ii) The diagonals of a rectangle are congruent.
∴ SQ = PR ...(v)
LN = MR ...(vi)
Now, MR = `1/2` PR ...(Point M is the midpoint of line PR.) ...(vii)
∴ LN = `1/2` PR ...[From (vi) and (vii)] ...(viii)
∴ LN = `1/2` SQ ...[From (vii) and (viii)]
APPEARS IN
RELATED QUESTIONS
In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively (see the given figure). Show that the line segments AF and EC trisect the diagonal BD.
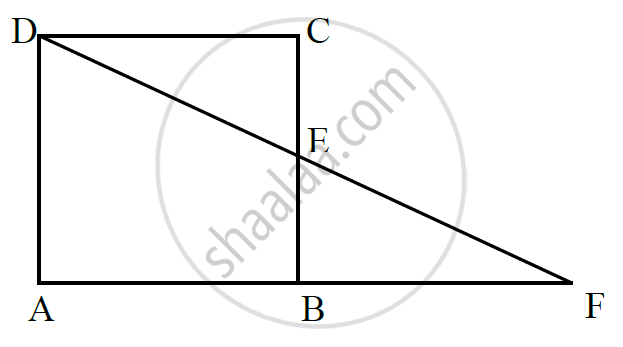
In below fig. ABCD is a parallelogram and E is the mid-point of side B If DE and AB when produced meet at F, prove that AF = 2AB.
In below Fig, ABCD is a parallelogram in which P is the mid-point of DC and Q is a point on AC such that CQ = `1/4` AC. If PQ produced meets BC at R, prove that R is a mid-point of BC.

In the adjacent figure, `square`ABCD is a trapezium AB || DC. Points M and N are midpoints of diagonal AC and DB respectively then prove that MN || AB.

Prove that the figure obtained by joining the mid-points of the adjacent sides of a rectangle is a rhombus.
In triangle ABC, AD is the median and DE, drawn parallel to side BA, meets AC at point E.
Show that BE is also a median.
In a parallelogram ABCD, M is the mid-point AC. X and Y are the points on AB and DC respectively such that AX = CY. Prove that:
(i) Triangle AXM is congruent to triangle CYM, and
(ii) XMY is a straight line.
In a parallelogram ABCD, E and F are the midpoints of the sides AB and CD respectively. The line segments AF and BF meet the line segments DE and CE at points G and H respectively Prove that: ΔHEB ≅ ΔHFC
In ΔABC, D and E are the midpoints of the sides AB and BC respectively. F is any point on the side AC. Also, EF is parallel to AB. Prove that BFED is a parallelogram.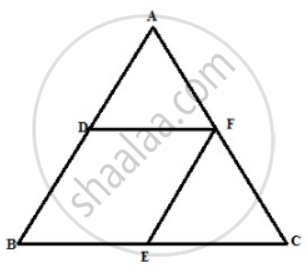
Remark: Figure is incorrect in Question
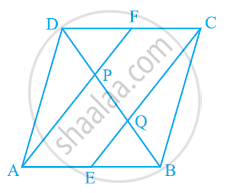
P and Q are the mid-points of the opposite sides AB and CD of a parallelogram ABCD. AQ intersects DP at S and BQ intersects CP at R. Show that PRQS is a parallelogram.
