Advertisements
Advertisements
Question
In the given figure, points X, Y, Z are the midpoints of side AB, side BC and side AC of ΔABC respectively. AB = 5 cm, AC = 9 cm and BC = 11 cm. Find the length of XY, YZ, XZ.

Solution
AB = 5 cm, AC = 9 cm and BC = 11 cm …(Given)
In ∆ABC,
Points X and Y are the midpoints of sides AB and BC respectively. ...(Given)
∴ XY = `1/2` AC ...(From midpoint theorem)
∴ XY = `1/2xx 9`
∴ XY = 4.5 cm
In ∆ABC,
Points Y and Z are the midpoints of sides BC and AC respectively. ...(Given)
∴ YZ = `1/2` AB ...(From midpoint theorem)
∴ YZ = `1/2xx 5`
∴ YZ = 2.5 cm
In ∆ABC,
Points X and Z are the midpoints of lines AB and AC respectively. ...(Given)
∴ XZ = `1/2` BC ...(From midpoint theorem)
∴ XZ = `1/2xx 11`
∴ XZ = 5.5 cm
APPEARS IN
RELATED QUESTIONS
In a ΔABC, BM and CN are perpendiculars from B and C respectively on any line passing
through A. If L is the mid-point of BC, prove that ML = NL.
In ΔABC, D, E, F are the midpoints of BC, CA and AB respectively. Find ∠FDB if ∠ACB = 115°.
In parallelogram PQRS, L is mid-point of side SR and SN is drawn parallel to LQ which meets RQ produced at N and cuts side PQ at M. Prove that M is the mid-point of PQ.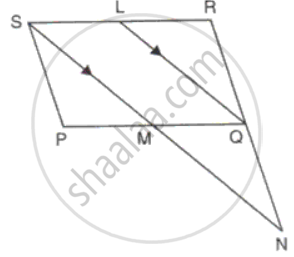
In a right-angled triangle ABC. ∠ABC = 90° and D is the midpoint of AC. Prove that BD = `(1)/(2)"AC"`.
In ΔABC, P is the mid-point of BC. A line through P and parallel to CA meets AB at point Q, and a line through Q and parallel to BC meets median AP at point R. Prove that: BC = 4QR
Side AC of a ABC is produced to point E so that CE = `(1)/(2)"AC"`. D is the mid-point of BC and ED produced meets AB at F. Lines through D and C are drawn parallel to AB which meets AC at point P and EF at point R respectively. Prove that: 4CR = AB.
In ΔABC, the medians BE and CD are produced to the points P and Q respectively such that BE = EP and CD = DQ. Prove that: Q A and P are collinear.
The figure formed by joining the mid-points of the sides of a quadrilateral ABCD, taken in order, is a square only if, ______.
E is the mid-point of a median AD of ∆ABC and BE is produced to meet AC at F. Show that AF = `1/3` AC.
E and F are respectively the mid-points of the non-parallel sides AD and BC of a trapezium ABCD. Prove that EF || AB and EF = `1/2` (AB + CD).
[Hint: Join BE and produce it to meet CD produced at G.]
