Advertisements
Advertisements
Question
E and F are respectively the mid-points of the non-parallel sides AD and BC of a trapezium ABCD. Prove that EF || AB and EF = `1/2` (AB + CD).
[Hint: Join BE and produce it to meet CD produced at G.]
Solution
Given: ABCD is a trapezium in which AB || CD. Also, E and F are respectively the mid-points of sides AD and BC.

Construction: Join BE and produce it to meet CD produced at G, also draw BD which intersects EF at O.
To prove: EF || AB and EF = `1/2` (AB + CD).
Proof: In ΔGCB, E and F are respectively the mid-points of BG and BC, then by mid-point theorem,
EF || GC
But GC || AB or CD || AB ...[Given]
∴ EF || AB
In ΔADB, AB || EO and E is the mid-point of AD.
Therefore by converse of mid-point theorem, O is mid-point of BD.
Also, EO = `1/2` AB ...(i)
In ΔBDC, OF || CD and O is the mid-point of BD.
∴ OF = `1/2` CD [By converse of mid-point theorem] ...(ii)
On adding equations (i) and (ii), we get
EO + OF = `1/2` AB + `1/2` CD
⇒ EF = `1/2` (AB + CD)
Hence proved.
APPEARS IN
RELATED QUESTIONS
ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
In below fig. ABCD is a parallelogram and E is the mid-point of side B If DE and AB when produced meet at F, prove that AF = 2AB.
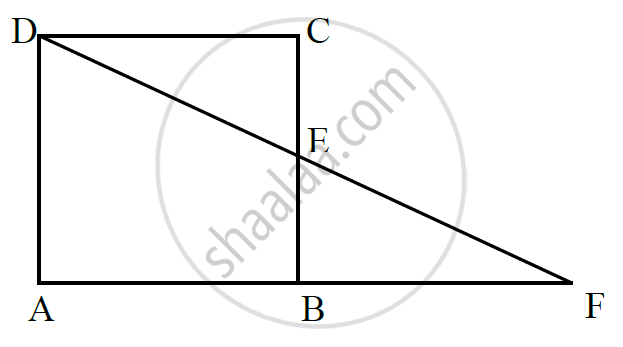
In Fig. below, triangle ABC is right-angled at B. Given that AB = 9 cm, AC = 15 cm and D,
E are the mid-points of the sides AB and AC respectively, calculate
(i) The length of BC (ii) The area of ΔADE.
Fill in the blank to make the following statement correct:
The figure formed by joining the mid-points of consecutive sides of a quadrilateral is
In parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P.
Prove that:
(i) BP = 2AD
(ii) O is the mid-point of AP.
In parallelogram ABCD, E and F are mid-points of the sides AB and CD respectively. The line segments AF and BF meet the line segments ED and EC at points G and H respectively.
Prove that:
(i) Triangles HEB and FHC are congruent;
(ii) GEHF is a parallelogram.
In ΔABC, D, E, F are the midpoints of BC, CA and AB respectively. Find DE, if AB = 8 cm
In the given figure, ABCD is a trapezium. P and Q are the midpoints of non-parallel side AD and BC respectively. Find: DC, if AB = 20 cm and PQ = 14 cm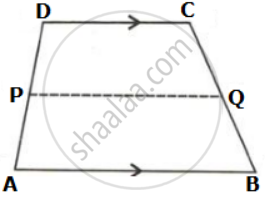
In ΔABC, D, E and F are the midpoints of AB, BC and AC.
Show that AE and DF bisect each other.
In a parallelogram ABCD, E and F are the midpoints of the sides AB and CD respectively. The line segments AF and BF meet the line segments DE and CE at points G and H respectively Prove that: ΔGEA ≅ ΔGFD
