Advertisements
Advertisements
Question
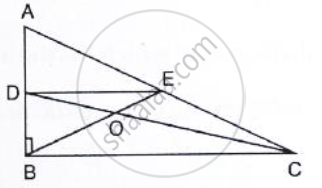
In Fig. below, triangle ABC is right-angled at B. Given that AB = 9 cm, AC = 15 cm and D,
E are the mid-points of the sides AB and AC respectively, calculate
(i) The length of BC (ii) The area of ΔADE.
Solution

In right ΔABC, ∠B = 90°
By using Pythagoras theorem
`AC^2 = AB^2+ BC^2`
⇒ `15^2 = 9^2 +BC^2`
⇒ BC =`sqrt(15^2 - 9^2)`
⇒ BC =`sqrt(225-81)`
⇒ BC =`sqrt144`
= 12cm
In ΔABC
D and E are midpoints of AB and AC
∴ DE || BC, DE = `1/2` BC [By midpoint theorem]
AD = OB = `(AB)/ 2= 9/2` = 4 . 5cm [ ∵ D is the midpoint of AB]
DE = `(BC)/2 = 12/2` = 6cm
Area of ΔADE = `1/2 xxAD xx DE `
= `1/2× 4 .5 × 6 = 13.5cm^2`
APPEARS IN
RELATED QUESTIONS
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that
- D is the mid-point of AC
- MD ⊥ AC
- CM = MA = `1/2AB`
ABCD is a square E, F, G and H are points on AB, BC, CD and DA respectively, such that AE = BF = CG = DH. Prove that EFGH is a square.
Let Abc Be an Isosceles Triangle in Which Ab = Ac. If D, E, F Be the Mid-points of the Sides Bc, Ca and a B Respectively, Show that the Segment Ad and Ef Bisect Each Other at Right Angles.
Fill in the blank to make the following statement correct:
The triangle formed by joining the mid-points of the sides of a right triangle is
In triangle ABC, P is the mid-point of side BC. A line through P and parallel to CA meets AB at point Q, and a line through Q and parallel to BC meets median AP at point R.
Prove that : (i) AP = 2AR
(ii) BC = 4QR
Show that the quadrilateral formed by joining the mid-points of the adjacent sides of a square is also a square.
In a parallelogram ABCD, E and F are the midpoints of the sides AB and CD respectively. The line segments AF and BF meet the line segments DE and CE at points G and H respectively Prove that: EGFH is a parallelogram.
D and E are the mid-points of the sides AB and AC of ∆ABC and O is any point on side BC. O is joined to A. If P and Q are the mid-points of OB and OC respectively, then DEQP is ______.
E is the mid-point of the side AD of the trapezium ABCD with AB || DC. A line through E drawn parallel to AB intersect BC at F. Show that F is the mid-point of BC. [Hint: Join AC]
P, Q, R and S are respectively the mid-points of sides AB, BC, CD and DA of quadrilateral ABCD in which AC = BD and AC ⊥ BD. Prove that PQRS is a square.
