Advertisements
Advertisements
प्रश्न
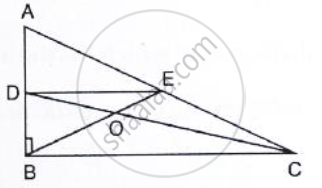
In Fig. below, triangle ABC is right-angled at B. Given that AB = 9 cm, AC = 15 cm and D,
E are the mid-points of the sides AB and AC respectively, calculate
(i) The length of BC (ii) The area of ΔADE.
उत्तर

In right ΔABC, ∠B = 90°
By using Pythagoras theorem
`AC^2 = AB^2+ BC^2`
⇒ `15^2 = 9^2 +BC^2`
⇒ BC =`sqrt(15^2 - 9^2)`
⇒ BC =`sqrt(225-81)`
⇒ BC =`sqrt144`
= 12cm
In ΔABC
D and E are midpoints of AB and AC
∴ DE || BC, DE = `1/2` BC [By midpoint theorem]
AD = OB = `(AB)/ 2= 9/2` = 4 . 5cm [ ∵ D is the midpoint of AB]
DE = `(BC)/2 = 12/2` = 6cm
Area of ΔADE = `1/2 xxAD xx DE `
= `1/2× 4 .5 × 6 = 13.5cm^2`
APPEARS IN
संबंधित प्रश्न
Show that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other.
In a ΔABC, BM and CN are perpendiculars from B and C respectively on any line passing
through A. If L is the mid-point of BC, prove that ML = NL.
In below Fig, ABCD is a parallelogram in which P is the mid-point of DC and Q is a point on AC such that CQ = `1/4` AC. If PQ produced meets BC at R, prove that R is a mid-point of BC.

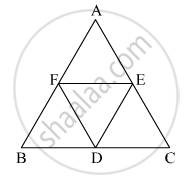
In the given figure, ΔABC is an equilateral traingle. Points F, D and E are midpoints of side AB, side BC, side AC respectively. Show that ΔFED is an equilateral traingle.
Prove that the figure obtained by joining the mid-points of the adjacent sides of a rectangle is a rhombus.
A parallelogram ABCD has P the mid-point of Dc and Q a point of Ac such that
CQ = `[1]/[4]`AC. PQ produced meets BC at R.

Prove that
(i)R is the midpoint of BC
(ii) PR = `[1]/[2]` DB
In the figure, give below, 2AD = AB, P is mid-point of AB, Q is mid-point of DR and PR // BS. Prove that:
(i) AQ // BS
(ii) DS = 3 Rs.

Prove that the straight lines joining the mid-points of the opposite sides of a quadrilateral bisect each other.
In a right-angled triangle ABC. ∠ABC = 90° and D is the midpoint of AC. Prove that BD = `(1)/(2)"AC"`.
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if ______.
