Advertisements
Advertisements
प्रश्न
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if ______.
विकल्प
PQRS is a rhombus
PQRS is a parallelogram
diagonals of PQRS are perpendicular
diagonals of PQRS are equal
उत्तर
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if diagonals of PQRS are equal.
Explanation:
Given, the quadrilateral ABCD is a rhombus.
So, sides AB, BC, CD and AD are equal.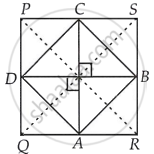
Now, in ΔPQS, we have
D and C are the mid-points of PQ and PS.
So, `DC = 1/2 QS` [By mid-point theorem] ...(i)
Similarly, in ΔPSR, `BC = 1/2 PR` [By mid-point theorem] ...(ii)
As BC = DC ...[Since, ABCD is a rhombus]
∴ `1/2 QS = 1/2 PR` ...[From equations (i) and (ii)]
⇒ QS = PR
Hence, diagonals of PQRS are equal.
APPEARS IN
संबंधित प्रश्न
ABCD is a parallelogram, E and F are the mid-points of AB and CD respectively. GH is any line intersecting AD, EF and BC at G, P and H respectively. Prove that GP = PH
Fill in the blank to make the following statement correct
The triangle formed by joining the mid-points of the sides of an isosceles triangle is
In the given figure, seg PD is a median of ΔPQR. Point T is the mid point of seg PD. Produced QT intersects PR at M. Show that `"PM"/"PR" = 1/3`.
[Hint: DN || QM]

In triangle ABC, M is mid-point of AB and a straight line through M and parallel to BC cuts AC in N. Find the lengths of AN and MN if Bc = 7 cm and Ac = 5 cm.
The diagonals of a quadrilateral intersect at right angles. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is rectangle.
A parallelogram ABCD has P the mid-point of Dc and Q a point of Ac such that
CQ = `[1]/[4]`AC. PQ produced meets BC at R.

Prove that
(i)R is the midpoint of BC
(ii) PR = `[1]/[2]` DB
In the figure, give below, 2AD = AB, P is mid-point of AB, Q is mid-point of DR and PR // BS. Prove that:
(i) AQ // BS
(ii) DS = 3 Rs.

If the quadrilateral formed by joining the mid-points of the adjacent sides of quadrilateral ABCD is a rectangle,
show that the diagonals AC and BD intersect at the right angle.
In the given figure, ABCD is a trapezium. P and Q are the midpoints of non-parallel side AD and BC respectively. Find: DC, if AB = 20 cm and PQ = 14 cm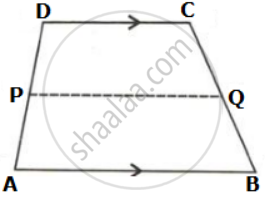
D, E and F are the mid-points of the sides BC, CA and AB, respectively of an equilateral triangle ABC. Show that ∆DEF is also an equilateral triangle.
