Advertisements
Advertisements
प्रश्न
The diagonals of a quadrilateral intersect at right angles. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is rectangle.
Advertisements
उत्तर
The figure is shown below
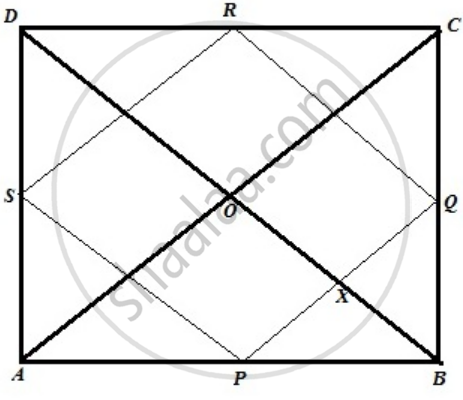
Let ABCD be a quadrilateral where P, Q, R, S are the midpoint of AB, BC, CD, DA. Diagonal AC and BD intersect at a right angle at point O. We need to show that PQRS is a rectangle
Proof:
From and ΔABC and ΔADC
2PQ = AC and PQ || AC …..(1)
2RS = AC and RS || AC …..(2)
From (1) and (2) we get,
PQ = RS and PQ || RS
Similarly, we can show that PS=RQ and PS || RQ
Therefore PQRS is a parallelogram.
Now PQ || AC, therefore ∠AOD = ∠PXO = 90° ...[ Corresponding angel ]
Again BD || RQ, therefore ∠PXO = ∠RQX = 90° ...[ Corresponding angel]
Similarly ∠QRS = ∠RSP = ∠SPQ = 90°
Therefore PQRS is a rectangle.
Hence proved.
APPEARS IN
संबंधित प्रश्न
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that
- D is the mid-point of AC
- MD ⊥ AC
- CM = MA = `1/2AB`
ABCD is a rhombus. EABF is a straight line such that EA = AB = BF. Prove that ED and FC when produced, meet at right angles.
ABCD is a kite having AB = AD and BC = CD. Prove that the figure formed by joining the
mid-points of the sides, in order, is a rectangle.
The following figure shows a trapezium ABCD in which AB // DC. P is the mid-point of AD and PR // AB. Prove that:
PR = `[1]/[2]` ( AB + CD)
In a triangle ABC, AD is a median and E is mid-point of median AD. A line through B and E meets AC at point F.
Prove that: AC = 3AF.
In the given figure, ABCD is a trapezium. P and Q are the midpoints of non-parallel side AD and BC respectively. Find: AB, if DC = 8 cm and PQ = 9.5 cm
In ΔABC, X is the mid-point of AB, and Y is the mid-point of AC. BY and CX are produced and meet the straight line through A parallel to BC at P and Q respectively. Prove AP = AQ.
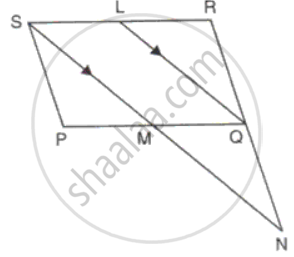
In ΔABC, D and E are the midpoints of the sides AB and AC respectively. F is any point on the side BC. If DE intersects AF at P show that DP = PE.
In ΔABC, D and E are the midpoints of the sides AB and BC respectively. F is any point on the side AC. Also, EF is parallel to AB. Prove that BFED is a parallelogram.
Remark: Figure is incorrect in Question
P, Q, R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.
