Advertisements
Advertisements
Question
In `square`ABCD, side BC < side AD in following figure. side BC || side AD and if side BA ≅ side CD then prove that ∠ABC ≅ ∠DCB.

Solution

Draw Seg BM ⊥ Seg AD such that A-M-D and Seg CN ⊥ AD so that A-N-D
Seg BC || Seg AD ...(Given)
∴ BM = CN ...(The distance between the lengths of the parallel sides of a parallelogram is equal.) ...(1)
In ∆BMA and ∆CND,
∠BMA = ∠CND = 90°
Hypotenuse BA ≅ Hypotenuse CD ...(Given)
Seg BM ≅ Seg CN ...[From (1)]
∴ ∆BMA ≅ ∆CND ...(Hypotenuse side test)
∴ ∠BAM ≅ ∠CDN ...(c.a.c.t)
That is, ∠BAD ≅ ∠CDA ...(A-M-D, A-N-D) ...(2)
Seg BC || Seg AD ...(Given)
and side AB is their transversal.
∴ ∠ABC + ∠BAD = 180° ...(interior angle) ...(3)
Seg BC || Seg AD ...(Given)
and side CD is their transversal.
∴ ∠DCB + ∠CDA = 180° ...(interior angle) ...(4)
∴ ∠ABC + ∠BAD = ∠DCB + ∠CDA ...[From (3) and (4)]
∴ ∠ABC + ∠CDA = ∠DCB + ∠CDA ...[From (2)]
∴ ∠ABC = ∠DCB
∴ ∠ABC ≅ ∠DCB
APPEARS IN
RELATED QUESTIONS
Explain how this figure is a trapezium. Which of its two sides are parallel?
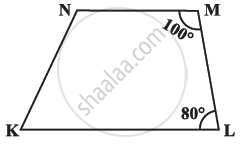
Find m∠C in the following figure if `bar(AB) || bar(DC)`
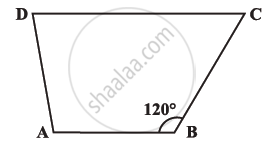
In the given figure CE || DB then the value of x° is
Which of the following figures satisfy the following property?
- Only one pair of sides are parallel.
In trapezium ABCD with AB || CD, if ∠A = 100°, then ∠D = ______.
Every trapezium is a parallelogram.
Of the four quadrilaterals-square, rectangle, rhombus and trapezium-one is somewhat different from the others because of its design. Find it and give justification.
In a quadrilateral HOPE, PS and ES are bisectors of ∠P and ∠E respectively. Give reason.
ABCD is a trapezium such that AB || CD, ∠A : ∠D = 2 : 1, ∠B : ∠C = 7 : 5. Find the angles of the trapezium.
Construct a trapezium RISK in which RI || KS, RI = 7 cm, IS = 5 cm, RK = 6.5 cm and ∠I = 60°.
