Topics
Number Systems
Number Systems
Polynomials
Algebra
Coordinate Geometry
Linear Equations in Two Variables
Geometry
Coordinate Geometry
Introduction to Euclid’S Geometry
Mensuration
Statistics and Probability
Lines and Angles
- Introduction to Lines and Angles
- Basic Terms and Definitions
- Intersecting Lines and Non-intersecting Lines
- Introduction to Parallel Lines
- Pairs of Angles
- Parallel Lines and a Transversal
- Angle Sum Property of a Triangle
Triangles
- Concept of Triangles
- Congruence of Triangles
- Criteria for Congruence of Triangles
- Properties of a Triangle
- Some More Criteria for Congruence of Triangles
- Inequalities in a Triangle
Quadrilaterals
- Concept of Quadrilaterals
- Properties of a Quadrilateral
- Types of Quadrilaterals
- Another Condition for a Quadrilateral to Be a Parallelogram
- Theorem of Midpoints of Two Sides of a Triangle
- Property: The Opposite Sides of a Parallelogram Are of Equal Length.
- Theorem: A Diagonal of a Parallelogram Divides It into Two Congruent Triangles.
- Theorem : If Each Pair of Opposite Sides of a Quadrilateral is Equal, Then It is a Parallelogram.
- Property: The Opposite Angles of a Parallelogram Are of Equal Measure.
- Theorem: If in a Quadrilateral, Each Pair of Opposite Angles is Equal, Then It is a Parallelogram.
- Property: The diagonals of a parallelogram bisect each other. (at the point of their intersection)
- Theorem : If the Diagonals of a Quadrilateral Bisect Each Other, Then It is a Parallelogram
Circles
Areas - Heron’S Formula
Surface Areas and Volumes
Statistics
Algebraic Expressions
Algebraic Identities
Area
Constructions
- Introduction of Constructions
- Basic Constructions
- Some Constructions of Triangles
Probability
Notes
Euclid’s fifth postulate is very significant in the history of mathematics. There are no intersection of lines will take place when the sum of the measures of the interior angles on the same side of the falling line is exactly 180°. There are several equivalent versions of this postulate. One of them is ‘Playfair’s Axiom’ as stated below:
‘For every line l and for every point P not lying on l, there exists a unique line m passing through P and parallel to l’. in the following fig . you can see that of all the lines passing through the point P , only line m is parallel to line l .
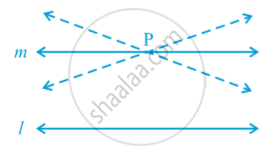
This result can also be stated in the following form:
Two distinct intersecting lines cannot be parallel to the same line.
All the attempts to prove Euclid’s fifth postulate using the first 4 postulates failed. But they led to the discovery of several other geometries, called non-Euclidean geometries.
Many mathematician including Euclid himself thought that fifth postulate is actually a theorem.
However, all attempts to prove the fifth postulate as a theorem have failed. but these efforts have led to the creation of several other geometries . They are called non-Euclidean geometries (Example : Spherical Geometry).

In spherical geometry, lines are not straight. They are parts of great circles (i.e., circles obtained by the intersection of a sphere and planes passing through the centre of the sphere).
In fig. the lines AN and BN (which are parts of great circles of a sphere) are perpendicular to the same line AB. But they are meeting each other, though the sum of the angles on the same side of line AB is not less than two right angles (in fact, it is 90° + 90° = 180°).
Also, note that the sum of the angles of the triangle NAB is greater than 180°, as ∠ A + ∠ B = 180°.
Thus, Euclidean geometry is valid only for the figures in the plane. On the curved surfaces, it fails.
