Advertisements
Advertisements
प्रश्न
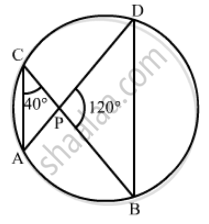
In the given figure, if ∠ACB = 40°, ∠DPB = 120°, find ∠CBD.
उत्तर
It is given that ∠ACB = 40° and ∠DPB = 120°

Construction: Join the point A and B
`angle ACB = angleADB = 40°` (Angle in the same segment)
Now in \[\bigtriangleup BDP\] we have
\[\angle DPB + \angle PBD + \angle BDP = 180° \]
\[ \Rightarrow 120° + \angle PBD + 40° = 180° \]
\[ \Rightarrow \angle PBD = 20° \]
Hence `angle CBD = 20° `
APPEARS IN
संबंधित प्रश्न
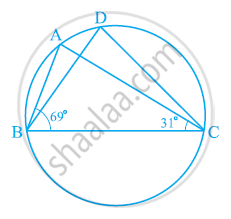
In the given figure, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.
If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figures.

In the given figure, O is the centre of the circle, BO is the bisector of ∠ABC. Show that AB = BC.
Prove that the angle in a segment shorter than a semicircle is greater than a right angle.
In the given figure, two congruent circles with centres O and O' intersect at A and B. If ∠AOB = 50°, then find ∠APB.

In the given figure, AB is a diameter of the circle such that ∠A = 35° and ∠Q = 25°, find ∠PBR.

In the given figure, P and Q are centres of two circles intersecting at B and C. ACD is a straight line. Then, ∠BQD =

A chord of a circle is equal to its radius. Find the angle subtended by this chord at a point in major segment.
