Advertisements
Advertisements
प्रश्न
In quadrilateral ABCD, diagonals AC and BD intersect at point E such that
AE : EC = BE : ED. Show that: ABCD is a trapezium.
उत्तर
Given, AE : EC = BE : ED
Draw EF || AB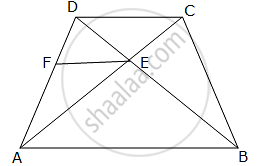
In ∆ABD, EF || AB
Using Basic Proportionality theorem,
`(DF)/(FA) = (DE)/(EB)`
But, given `(DE)/(EB) = (CE)/(EA)`
∴ `(DF)/(FA) = (CE)/(EA)`
Thus, in ∆DCA, E and F are points on CA and DA respectively such that
`(DF)/(FA) = (CE)/(EA)`
Thus, by converse of Basic proportionality theorem, FE || DC.
But, FE || AB.
Hence, AB || DC.
Thus, ABCD is a trapezium.
APPEARS IN
संबंधित प्रश्न
State, true or false:
Two isosceles triangles are similar, if an angle of one is congruent to the corresponding angle of the other.
In a trapezium ABCD, side AB is parallel to side DC; and the diagonals AC and BD intersect each other at point P. Prove that : PA x PD = PB x PC.
In the given figure, AB || DC, BO = 6 cm and DQ = 8 cm; find: BP × DO.

Through the mid-point M of the side CD of a parallelogram ABCD, the line BM is drawn intersecting diagonal AC in L and AD produced in E. Prove that: EL = 2BL.
In the given figure, AB and DE are perpendiculars to BC.
Prove that : ΔABC ~ ΔDEC
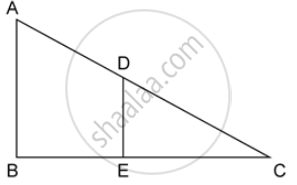
In the given figure, AB and DE are perpendiculars to BC.
If AB = 6 cm, DE = 4 cm and AC = 15 cm. Calculate CD.

In the adjoining figure, ΔACB ∼ ∆APQ. If BC = 10 cm, PQ = 5 cm, BA = 6.5 cm and AP = 2.8 cm find the area (∆ACB) : area (∆APQ).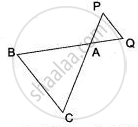
Two isosceles triangle have equal vertical angles and their areas are in the ratio of 36 : 25. Find the ratio between their corresponding heights.
Triangles ABC and DEF are similar.
If area (ΔABC) = 16 cm2, area (ΔDEF) = 25 cm2 and BC = 2·3 cm find EF.
Triangles ABC and DEF are similar.
If area (ΔABC) = 9 cm2, area (ΔDEF) = 64 cm2 and BC = 5·1 cm find AB.
