Advertisements
Advertisements
प्रश्न
In the figure, PQRS and PTVS are two cyclic quadrilaterals, If ∠QRS = 100°, then ∠TVS =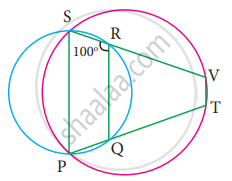
विकल्प
80°
100°
70°
90°
उत्तर
100°
Explanation;
Hint:
∠SPQ = 180° – 100° ...(Opposite angles of a cyclic quadrilateral PQRS)
= 80°
In the cyclic quadrilateral PVTS,
∠V = 180° – 80° = 100° ...(Opposite angles of a cyclic quadrilateral)
APPEARS IN
संबंधित प्रश्न
The measure of angles of a hexagon are x°, (x − 5)°, (x − 5)°, (2x − 5)°, (2x − 5)°, (2x + 20)°. Find the value of x.
PQRSTU is a regular hexagon. Determine each angle of ΔPQT.
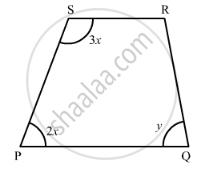
In the given figure, PQRS is an isosceles trapezium. Find x and y.
Mark the correct alternative in each of the following:
The opposite sides of a quadrilateral have
ABCDE is a regular pentagon. The bisector of angle A of the pentagon meets the side CD in point M. Show that ∠AMC = 90°.
If angles A, B, C and D of the quadrilateral ABCD, taken in order, are in the ratio 3 : 7 : 6 : 4, then ABCD is a ______.
Using the information given, name the right angles in part of figure:
AC ⊥ BD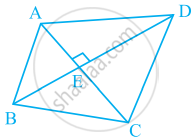
Can we have two obtuse angles whose sum is a complete angle? Why or why not?
Draw a rough sketch of a quadrilateral KLMN. State two pairs of opposite angles.
Investigate :
Use strips and fasteners to make a triangle and a quadrilateral.
Try to push inward at any one vertex of the triangle. Do the same to the quadrilateral. Is the triangle distorted? Is the quadrilateral distorted? Is the triangle rigid?
Why is it that structures like electric towers make use of triangular shapes and not quadrilaterals?
