Advertisements
Advertisements
प्रश्न
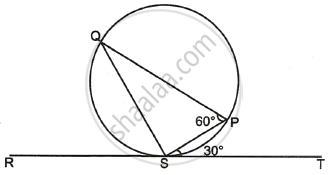
In the given diagram RT is a tangent touching the circle at S. If ∠PST = 30° and ∠SPQ = 60°, then ∠PSQ is equal to ______.
विकल्प
40°
30°
60°
90°
उत्तर
In the given diagram RT is a tangent touching the circle at S. If ∠PST = 30° and ∠SPQ = 60°, then ∠PSQ is equal to 90°.
Explanation:

∠PQS = ∠PST ...(∠S in alternate segment)
= 30°
In ΔPQS,
∠PQS + ∠PSQ + ∠SPQ = 180°
30° + ∠PSQ + 60° = 180°
∠PSQ = 180° – 90°
= 90°
APPEARS IN
संबंधित प्रश्न
A tangent to a circle intersects it in ______ point (s).
A line intersecting a circle in two points is called a ______.
If the angle between two tangents drawn from an external point P to a circle of radius a and centre O, is 60°, then find the length of OP
In fig., AB and DC are two chords of a circle with centre O. these chords when produced meet at P. if PB = Bern, BA = 7cm and PO = 14.5cm, find the radius of the circle.

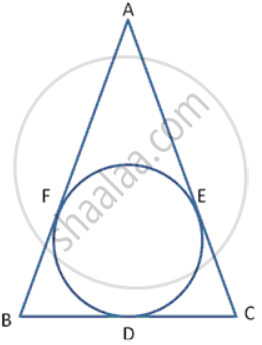
In following figure , the incircle of Δ ABC , touches the sides BC , CA and AB at D , E and F respectively. Show AF + BD + CE = AE + BF + CD
In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If angle ACO = 30°, find: angle AOB

In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find:
- AB.
- the length of tangent PT.

At one end A of a diameter AB of a circle of radius 5 cm, tangent XAY is drawn to the circle. The length of the chord CD parallel to XY and at a distance 8 cm from A is ______
The distance between two tangents parallel to each other of a circle is 13 cm. Find the radius of the circle.
If O is centre of a circle and Chord PQ makes an angle 50° with the tangent PR at the point of contact P, then the angle subtended by the chord at the centre is ______.