Advertisements
Advertisements
प्रश्न
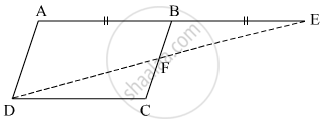
In the given figure, `square`ABCD is a parallelogram. Point E is on the ray AB such that BE = AB then prove that line ED bisects seg BC at point F.
उत्तर
`square`ABCD is a parallelogram. ...[Given]
∴ seg AB ≅ seg DC ...(i) [Opposite angles of a parallelogram]
seg AB ≅ seg BE ...(ii) [Given]
seg DC ≅ seg BE ...(iii) [From (i) and (ii)]
side DC || side AB ...[Opposite sides of a parallelogram]
i.e. side DC || seg AE and seg DE is their transversal. ...[A-B-E]
∴ ∠CDE ≅ ∠AED
∴ ∠CDF ≅ ∠BEF ...(iv) [D-F-E, A-B-E]
In ∆DFC and ∆EFB,
seg DC = seg EB ...[From (iii)]
∠CDF ≅ ∠BEF ...[From (iv)]
∠DFC ≅ ∠EFB ...[Vertically opposite angles]
∴ ∆DFC ≅ ∆EFB ...[SAA test]
∴ FC ≅ FB ...[c.s.c.t]
∴ Line ED bisects seg BC at point F.
APPEARS IN
संबंधित प्रश्न
Can a quadrilateral ABCD be a parallelogram if ∠D + ∠B = 180°?
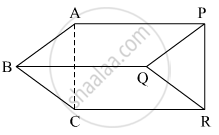
In the adjacent figure, if seg AB || seg PQ, seg AB ≅ seg PQ, seg AC || seg PR, seg AC ≅ seg PR then prove that, seg BC || seg QR and seg BC ≅ seg QR.
The given figure shows parallelogram ABCD. Points M and N lie in diagonal BD such that DM = BN.
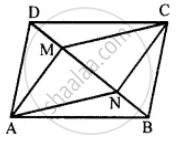
Prove that:
(i) ∆DMC = ∆BNA and so CM = AN
(ii) ∆AMD = ∆CNB and so AM CN
(iii) ANCM is a parallelogram.
In the given figure, AB || EC, AB = AC and AE bisects ∠DAC. Prove that:
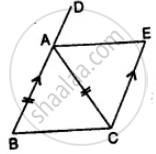
- ∠EAC = ∠ACB
- ABCE is a parallelogram.
In the given figure, ABCD and BDCE are parallelograms with common base DC. If BC ⊥ BD, then ∠BEC = ______.
All rectangles are parallelograms.
ABCD is a parallelogram. Find the value of x, y and z.

ABCD is a parallelogram. Points P and Q are taken on the sides AB and AD respectively and the parallelogram PRQA is formed. If ∠C = 45°, find ∠R.
Construct a parallelogram HOME with HO = 6 cm, HE = 4 cm and OE = 3 cm.
Draw a rough figure of a quadrilateral that is not a parallelogram but has exactly two opposite angles of equal measure.
