Advertisements
Advertisements
प्रश्न
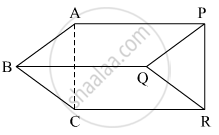
In the adjacent figure, if seg AB || seg PQ, seg AB ≅ seg PQ, seg AC || seg PR, seg AC ≅ seg PR then prove that, seg BC || seg QR and seg BC ≅ seg QR.
उत्तर
Given: seg AB || seg PQ, seg AB ≅ seg PQ,
seg AC || seg PR, seg AC ≅ seg PR
To prove: seg BC || seg QR, seg BC ≅ seg QR
Proof:
In `square`ABQP,
seg AB || seg PQ
seg AB ≅ seg PQ ...(Given)
∴ `square`ABQP is a parallelogram. ...(A quadrilateral is a parallelogram if a pair of its opposite sides is parallel and congruent)
∴ segAP || segBQ ...(i)
∴ seg AP ≅ seg BQ ...(ii) ...(Opposite sides of a parallelogram)
In `square`ACRP,
seg AC || seg PR
seg AC ≅ seg PR ...(Given)
∴ `square`ACRP is a parallelogram. ...(A quadrilateral is a parallelogram if a pair of its opposite sides is parallel and congruent)
∴ seg AP || seg CR ...(iii)
∴ seg AP ≅ seg CR ...(iv) ...(Opposite sides of a parallelogram)
In `square`BQRC,
seg BQ || seg CR ...[From (i) and (iii)]
seg BQ ≅ seg CR ...[From (ii) and (iv)]
∴ `square`BQRC is a parallelogram. ...(A quadrilateral is a parallelogram if a pair of its opposite sides is parallel and congruent)
∴ seg BC || seg QR
∴ seg BC ≅ seg QR ...(Opposite sides of parallelogram)
APPEARS IN
संबंधित प्रश्न
Given a parallelogram ABCD. Complete each statement along with the definition or property used.

- AD = ______
- ∠DCB = ______
- OC = ______
- m∠DAB + m∠CDA = ______
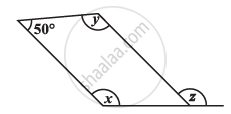
Consider the given parallelogram. Find the values of the unknowns x, y, z.
Can a quadrilateral ABCD be a parallelogram if ∠A = 70° and ∠C = 65°?
The following figure GUNS is a parallelogram. Find x and y. (Lengths are in cm)

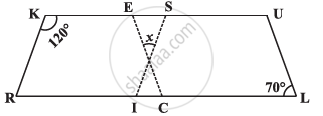
In the above figure both RISK and CLUE are parallelograms. Find the value of x.
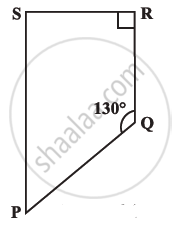
Find the measure of ∠P and ∠S, if `bar(SP) || bar(RQ)` in the following figure. (If you find m∠R, is there more than one method to find m∠P?).
PQRS is a parallelogram whose diagonals intersect at M.
If ∠PMS = 54°, ∠QSR = 25° and ∠SQR = 30° ; find :
(i) ∠RPS
(ii) ∠PRS
(iii) ∠PSR.
In parallelogram ABCD, E is the mid-point of AD and F is the mid-point of BC. Prove that BFDE is a parallelogram.
In parallelogram ABCD, X and Y are midpoints of opposite sides AB and DC respectively. Prove that:
(i) AX = YC
(ii) AX is parallel to YC
(iii) AXCY is a parallelogram.
ABCD is a parallelogram. Points P and Q are taken on the sides AB and AD respectively and the parallelogram PRQA is formed. If ∠C = 45°, find ∠R.
