Advertisements
Advertisements
प्रश्न
In the given figure, PA is a tangent to the circle drawn from the external point P and PBC is the secant to the circle with BC as diameter. If ∠AOC = 130°, then find the measure of ∠APB, where O is the centre of the circle.

उत्तर
In ΔAPO, ∠AOC is the exterior angle
∴ From exterior angle property.
∠AOC = ∠PAO + ∠APB ...`{{:(∵ ∠AOC = 130^circ ("Given")),(∠PAO = 90^circ "(radius and tangent"),("are" ⊥ "to each other at the point of contact)"):}`
`\implies` 130° = 90° + ∠APB
`\implies` ∠APB = 130° – 90°
`\implies` ∠APB = 40°
संबंधित प्रश्न
In the figure given below, PQ is a chord of a circle with centre O and PT is a tangent. If ∠QPT = 60°, find ∠PRQ.

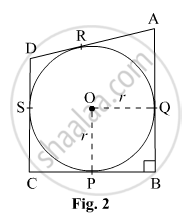
In Fig.2, a circle with centre O is inscribed in a quadrilateral ABCD such that, it touches the sides BC, AB, AD and CD at points P, Q, R and S respectively, If AB = 29 cm, AD = 23 cm, ∠B = 90° and DS = 5 cm, then the radius of the circle (in cm.) is:

In the figure, point Q is the
point of contact. If PQ = 12,
PR = 8 then find PS.
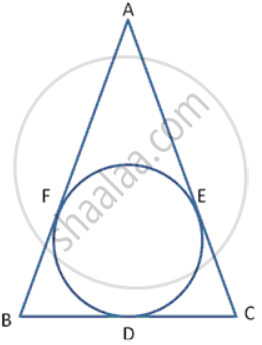
In following figure , the incircle of Δ ABC , touches the sides BC , CA and AB at D , E and F respectively. Show AF + BD + CE = AE + BF + CD
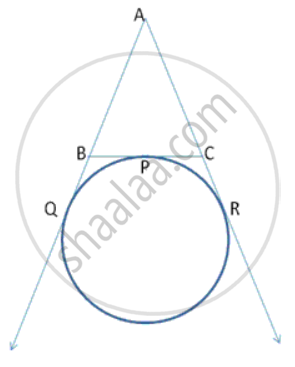
In following fig., a circle is touching the side BC of Δ ABC at P and AB and AC produced at Q and R respectively. Prove that AQ is half the perimeter of Δ ABC.
In a square ABCD, its diagonal AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and bisector of angle ABD meets AC at N and AM at L. Show that - ALOB is a cyclic quadrilateral.
Construct a tangent to a circle with centre O and radius 3.5 cm at a point P on it.
In the figure, if PA and PB are tangents to the circle with centre O such that ∠APB = 50°, then ∠OAB is equal to ______.

The distance between two parallel tangents of a circle of radius 4 cm is ______
Tangents from an external point to a circle are ______
