Advertisements
Advertisements
प्रश्न
Inside a square field of side 44m, a square flower bed is prepared leaving a graved path all round the flower bed. The total cost of laying the flower bed at Rs.25per sq m. and gravelling the path at Rs.120per sq m. is Rs.80320. Find the width of the gravel path.
उत्तर
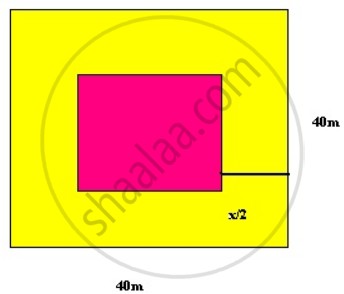
Let the width of the gravel path = `x/(2)"m"`
Now, Area of flower bed (shown in Pink) x 25 + Area of gravel path(Shown in Yellow) = Rs.80320
i.e (44 - x)2 x 25 + [442 - (44 - x)2 x 120 = 80320
(44 -x)2 x 25 + 442 x 120 - (44 - x)2 x 120 = 80320
(44 - x)2 x (25 - 120) + 442 x 120 = 80320
(44 - x)2 x (-95) + 1936 x 120 = 80320
(44 - x)2 x (-19) + 1936 x 24 = 16064
46464 - 1664 = 19(44 - x)2
30400 = 19(44 - x)2
(44 - x)2 = 16000
44 - x = 40
x = 4
the width of the grave path = `x/(2)` = 2m.
APPEARS IN
संबंधित प्रश्न
ABCD is a rectangle with ∠ABD = 40°. Determine ∠DBC .
State, 'true' or 'false'
If two adjacent sides of a parallelogram are equal, it is a rhombus.
In a square ABCD, diagonals meet at O. P is a point on BC such that OB = BP.
Show that:
- ∠POC = `[ 22 ( 1°)/( 2 ) ]`
- ∠BDC = 2 ∠POC
- ∠BOP = 3 ∠CPO
The diagonals of a parallelogram ABCD intersect at O. A line through O meets AB in P and CD in Q. Show that
(a) Area of APQD = `(1)/(2)` area of || gm ABCD
(b) Area of APQD = Area of BPQC
In the given figure, BC ∥ DE.
(a) If area of ΔADC is 20 sq. units, find the area of ΔAEB.
(b) If the area of ΔBFD is 8 square units, find the area of ΔCEF
If the medians of a ΔABBC intersect at G, show that ar(ΔAGB) = ar(ΔAGC) = ar(ΔBGC) = `(1)/(3)"ar(ΔABC)"`.
In the given figure, ABC is a triangle and AD is the median.
If E is the midpoint of the median AD, prove that: Area of ΔABC = 4 × Area of ΔABE
Find the area of each of the following figure:
Find the area of each of the following figure:
One side of a parallelogram is 12cm and the altitude corresponding to i is 8cm. If the length of the altitude corresponding to its adjacent side is 16cm, find the length of the adjacent side.
A rectangular hall of 40m by 24m is covered with carpets of size 6m x 4m. Find the number of carpets required to cover the hall.
The area of a square garden is equal to the area of a rectangular plot of length 160m and width 40m. Calculate the cost of fencing the square garden at Rs.12per m.
Find the perimeter and area of a square whose diagonal is `5sqrt(2)"cm"`. Give your answer correct to two decimal places if `sqrt(2)` = 1.414.
Find the perimeter of a rhombus whose diagonals are 24cm and 10cm.
In quadrilateral ABCD, ∠A + ∠D = 180º. What special name can be given to this quadrilateral?
ABCD is a trapezium in which AB || DC and ∠A = ∠B = 45º. Find angles C and D of the trapezium.
The opposite sides of a rectangle are equal in length.
Give reason for the following :
A square can be thought of as a special rectangle.
Give reason for the following :
Squares, rectangles, parallelograms are all quadrilaterals.
