Advertisements
Advertisements
प्रश्न
Is the area of the largest circle that can be drawn inside a rectangle of length a cm and breadth b cm (a > b) is πb2 cm2? Why?
उत्तर
The largest circle that can be drawn inside a rectangle is possible when rectangle becomes a square.
∴ Diameter of the circle = Breadth of the rectangle = b
∴ Radius of the circle = `"b"/2`
Hence area of the circle = πr2 = `π("b"/2)^2`
APPEARS IN
संबंधित प्रश्न
Find the length of the hypotenuse of an isosceles right-angled triangle whose area is `200^2` cm . Also, find its perimeter
In the following figure, ABCD is a rectangle, having AB = 20 cm and BC = 14 cm. Two sectors of 180° have been cut off. Calculate:
the length of the boundary of the shaded region.
In the following figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the (i) quadrant OACB (ii) shaded region.

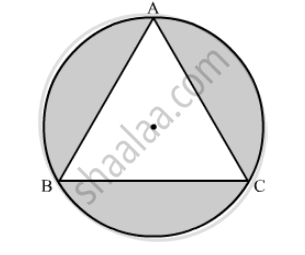
In the following figure, an equilateral triangle ABC of side 6 cm has been inscribed in a circle. Find the area of the shaded region. (Take π = 3.14).
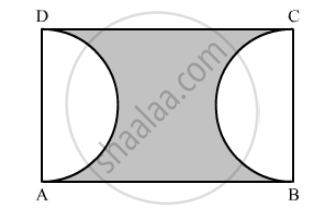
Two circular pieces of equal radii and maximum area, touching each other are cut out from a rectangular card board of dimensions 14 cm × 7 cm. Find the area of the remaining card board. (Use π = 22/7).
If the perimeter of a sector of a circle of radius 6.5 cm is 29 cm, then its area is
If the area of a sector of a circle bounded by an arc of length 5π cm is equal to 20π cm2, then its radius is
The area of a sector whose perimeter is four times its radius r units, is
On increasing the diameter of a circle by 40%, its area will be increased by
What is the diameter of a circle whose area is equal to the sum of the areas of two circles of radii 40cm and 9cm?
