Advertisements
Advertisements
प्रश्न
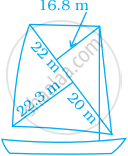
Most of the sailboats have two sails, the jib and the mainsail. Assume that the sails are triangles. Find the total area sail of the sailboats to the nearest tenth.
उत्तर
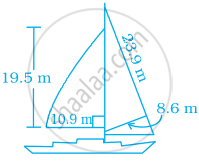
In sailboat,
Area of a triangle = `1/2` × Base × Height
In ΔABC, ∠B = 90°, base (BC) = 10.9 m and height (AB) = 19.5 m
Area of ΔABC = `1/2 xx 10.9 xx 19.5`
= `212.55/2`
= 106.275 m2
In another triangular part,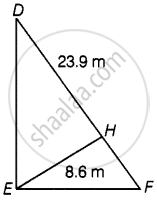
Area of ΔDEF = `1/2 xx DF xx EH`
= `1/2 xx 23.9 xx 8.6`
= `205.54/2`
= 102.77 m2
Area of sailboat = 106.275 + 102.77 = 209.045 m2
APPEARS IN
संबंधित प्रश्न
A thin metal iron-sheet is rhombus in shape, with each side 10 m. If one of its diagonals is 16 m, find the cost of painting both sides at the rate of ₹ 6 per m2. Also, find the distance between the opposite sides of this rhombus.
The area of a rhombus is equal to the area of a triangle. If the base ∆ is 24 cm, its corresponding altitude is 16 cm and one of the diagonals of the rhombus is 19.2 cm. Find its other diagonal.
Find the area of a rhombus whose base is 14 cm and height is 9 cm.
The area of the rhombus with side 4 cm and height 3 cm is
The area of the rhombus is 128 sq.cm and the length of one diagonal is 32 cm. The length of the other diagonal is
The angle between the diagonals of a rhombus is
A man has to build a rhombus shaped swimming pool. One of the diagonal is 13 m and the other is twice the first one. Then find the area of the swimming pool and also find the cost of cementing the floor at the rate of ₹ 15 per sq.cm
What is the area of the rhombus ABCD below if AC = 6 cm and BE = 4 cm?
The walls and ceiling of a room are to be plastered. The length, breadth and height of the room are 4.5 m, 3 m, and 350 cm respectively. Find the cost of plastering at the rate of Rs 8 per m2.
Most of the sailboats have two sails, the jib and the mainsail. Assume that the sails are triangles. Find the total area sail of the sailboats to the nearest tenth.